Advertisements
Advertisements
Question
Find the thermo-emf developed in a copper-silver thermocouple when the junctions are kept at 0°C and 40°C. Use the data given in the following table.
| Metal with lead (Pb) |
a `mu V"/"^oC` |
b `muV"/("^oC)` |
| Aluminium | -0.47 | 0.003 |
| Bismuth | -43.7 | -0.47 |
| Copper | 2.76 | 0.012 |
| Gold | 2.90 | 0.0093 |
| Iron | 16.6 | -0.030 |
| Nickel | 19.1 | -0.030 |
| Platinum | -1.79 | -0.035 |
| Silver | 2.50 | 0.012 |
| Steel | 10.8 | -0.016 |
Solution
Difference in temperature, θ = 40°C
Emf, Ecs = acsθ + `1/2` bcsθ2 ...........(1)
acs = [2.76 − (−43.7) μV
= 46.46 μV/°C
bcs = [0.012 − (−0.47) μV/°C
= 0.482 μV/°C2
Putting this value in eq. (1), we get:-
Ecs = 46.46 × 10−6 × 40 + `1/2` × 0.482 × 10−6 × (40)2
= 1.04 × 10−5 V
APPEARS IN
RELATED QUESTIONS
At room temperature (27.0°C) the resistance of a heating element is 100 Ω. What is the temperature of the element if the resistance is found to be 117 Ω, given that the temperature coefficient of the material of the resistor is 1.70 × 10−4 °C−1.
The order of coloured rings in a carbon resistor is red, yellow, blue and silver. The resistance of the
carbon resistor is:
a) 24 x 106 Ω ± 5%
b) 24 x 106 Ω ± 10%
c) 34 x 104 Ω ± 10%
d) 26 x 104 Ω ± 5%
Consider a circuit containing an ideal battery connected to a resistor. Do "work done by the battery" and "the thermal energy developed" represent two names of the same physical quantity?
Is work done by a battery always equal to the thermal energy developed in electrical circuit? What happens if a capacitor is connected in the circuit?
Sometimes it is said that "heat is developed" in a resistance when there is an electric current in it. Recall that heat is defined as the energy being transferred due to temperature difference. Is the statement in quotes technically correct?
Two resistors R and 2R are connected in series in an electric circuit. The thermal energy developed in R and 2R are in the ratio ______________ .
The resistance of an iron wire and a copper wire at 20°C are 3.9 Ω and 4.1 Ω, respectively. At what temperature will the resistance be equal? Temperature coefficient of resistivity for iron is 5.0 × 10–3 K–1 and for copper, it is 4.0 × 10–3 K–1. Neglect any thermal expansion.
Is neutral temperature always the arithmetic mean of the inversion temperature and the temperature of the cold junction? Does the unit of temperature have an effect in deciding this question?
As temperature increases, the viscosity of liquids decreases considerably. Will this decrease the resistance of an electrolyte as the temperature increases?
Consider the following statements regarding a thermocouple.
(A) The neutral temperature does not depend on the temperature of the cold junction.
(B) The inversion temperature does not depend on the temperature of the cold junction.
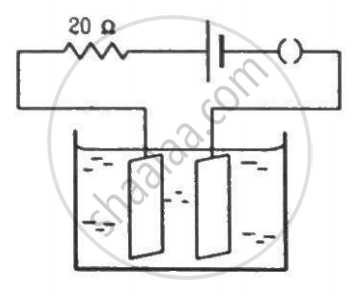
The figure shows an electrolyte of AgCl through which a current is passed. It is observed that 2.68 g of silver is deposited in 10 minutes on the cathode. Find the heat developed in the 20 Ω resistor during this period. Atomic weight of silver is 107.9 g/mol−1.
Find the neutral temperature and inversion temperature of a copper-iron thermocouple if the reference junction is kept at 0°C. Use the data given in the following table.
| Metal with lead (Pb) |
a `mu V"/"^oC` |
b `muV"/("^oC)` |
| Aluminium | -0.47 | 0.003 |
| Bismuth | -43.7 | -0.47 |
| Copper | 2.76 | 0.012 |
| Gold | 2.90 | 0.0093 |
| Iron | 16.6 | -0.030 |
| Nickel | 19.1 | -0.030 |
| Platinum | -1.79 | -0.035 |
| Silver | 2.50 | 0.012 |
| Steel | 10.8 | -0.016 |
Define temperature coefficient of resistance of the material of a conductor.
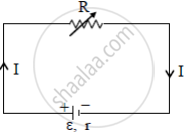
A variable resistor R is connected across a cell of emf ε and internal resistance r as shown in the figure. Draw a plot showing the variation of
(i) Terminal voltage V and
(ii) the current I, as a function of R.
The higher and lower fixed points on a thermometer are separated by 160 mm. When the length of the mercury thread above the lower point is 40 mm, the temperature reading would be :
Water at 10°C enters into a geyser. The water drawn out from the geyser has a temperature of 60°C and the rate of outflow of water is 18 kg/hr. The rating of the geyser is :
Temperature dependence of resistivity ρ(T) of semiconductors, insulators and metals is significantly based on the following factors:
- number of charge carriers can change with temperature T.
- time interval between two successive collisions can depend on T.
- length of material can be a function of T.
- mass of carriers is a function of T.
