Advertisements
Advertisements
Question
The resistance of an iron wire and a copper wire at 20°C are 3.9 Ω and 4.1 Ω, respectively. At what temperature will the resistance be equal? Temperature coefficient of resistivity for iron is 5.0 × 10–3 K–1 and for copper, it is 4.0 × 10–3 K–1. Neglect any thermal expansion.
Solution
Given:-
Resistance RFe of the iron wire at 20°C = 3.9 Ω
Resistance RCu of the copper wire at 20°C = 4.1 Ω
Temperature coefficient αFe for iron = 5.0 × 10–3 K–1
Temperature coefficient αCu for copper = 4.0 × 10–3 K–1
Let
The temperature at which the resistance be equal = T
Resistance of iron wire at T °C = RFe'
Resistance of copper wire at T °C = RCu'
We know:-
\[R = R_0 (1 + \alpha ∆ T)\]
Here, ΔT = T - 20
\[\Rightarrow R_{Fe} ' = R_{Fe} \left[ 1 + \alpha_{Fe} \left( T - 20 \right) \right]\]
\[ R_{Cu} ' = R_{Cu} \left[ 1 + \alpha_{Cu} \left( T - 20 \right) \right]\]
\[ R_{Fe} ' = R_{Cu} '\]
\[ \Rightarrow R_{Fe} \left[ 1 + \alpha_{Fe} \left( T - 20 \right) \right] = R_{Cu} \left[ 1 + \alpha_{Cu} \left( T - 20 \right) \right]\]
\[ \Rightarrow 3 . 9 \left[ 1 + 5 \times {10}^{- 3} \left( T - 20 \right) \right] = 4 . 1 \left[ 1 + 4 \times {10}^{- 3} \left( T - 20 \right) \right]\]
\[ \Rightarrow 3 . 9 + 3 . 9 \times 5 \times {10}^{- 3} \left( T - 20 \right) = 4 . 1 + 4 . 1 \times 4 \times {10}^{- 3} \left( T - 20 \right)\]
\[ \Rightarrow 4 . 1 \times 4 \times {10}^{- 3} \left( T - 20 \right) - 3 . 9 \times 5 \times {10}^{- 3} \left( T - 20 \right) = - 4 . 1 + 3 . 9\]
\[ \Rightarrow 16 . 4 \left( T - 20 \right) - 19 . 5 \left( T - 20 \right) = - 0 . 2 \times {10}^3 \]
\[ \Rightarrow \left( T - 20 \right)\left( - 3 . 1 \right) = - 0 . 2 \times {10}^3 \]
\[ \Rightarrow T - 20 = 64 . 5\]
\[ \Rightarrow T = 84 . 5^\circ K\]
APPEARS IN
RELATED QUESTIONS
Draw labelled graphs to show how electrical resistance varies with temperature for:
1) a metallic wire.
2) a piece of carbon
Is work done by a battery always equal to the thermal energy developed in electrical circuit? What happens if a capacitor is connected in the circuit?
A non-ideal battery is connected to a resistor. Is work done by the battery equal to the thermal energy developed in the resistor? Will your answer change if the battery is ideal?
As the temperature of a metallic resistor is increased, the product of its resistivity and conductivity ____________ .
When a current passes through a resistor, its temperature increases. Is it an adiabatic process?
An electric kettle used to prepare tea, takes 2 minutes to boil 4 cups of water (1 cup contains 200 cc of water) if the room temperature is 25°C. (a) If the cost of power consumption is Re 1.00 per unit (1 unit = 1000 watt-hour), calculate the cost of boiling 4 cups of water. (b) What will be the corresponding cost if the room temperature drops to 5°C?
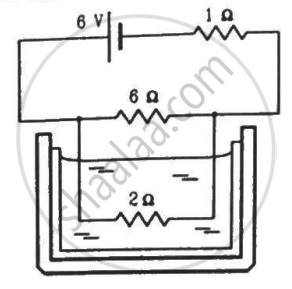
The 2.0 Ω resistor shown in the figure is dipped into a calorimeter containing water. The heat capacity of the calorimeter together with water is 2000 J K−1. (a) If the circuit is active for 15 minutes, what would be the rise in the temperature of the water? (b) Suppose the 6.0 Ω resistor gets burnt. What would be the rise in the temperature of the water in the next 15 minutes?
Find the thermo-emf developed in a copper-silver thermocouple when the junctions are kept at 0°C and 40°C. Use the data given in the following table.
| Metal with lead (Pb) |
a `mu V"/"^oC` |
b `muV"/("^oC)` |
| Aluminium | -0.47 | 0.003 |
| Bismuth | -43.7 | -0.47 |
| Copper | 2.76 | 0.012 |
| Gold | 2.90 | 0.0093 |
| Iron | 16.6 | -0.030 |
| Nickel | 19.1 | -0.030 |
| Platinum | -1.79 | -0.035 |
| Silver | 2.50 | 0.012 |
| Steel | 10.8 | -0.016 |
Define temperature coefficient of resistance of the material of a conductor.
A metallic wire has a resistance of 3.0 Ω at 0°C and 4.8 Ω at 150°C. Find the temperature coefficient of resistance of its material.
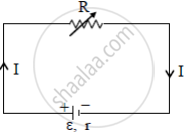
A variable resistor R is connected across a cell of emf ε and internal resistance r as shown in the figure. Draw a plot showing the variation of
(i) Terminal voltage V and
(ii) the current I, as a function of R.
An electrical cable of copper has just one wire of radius 9 mm. Its resistance is 5 ohm. This single copper wire of the cable is replaced by 6 different well insulated copper wires each of radius 3 mm. The total resistance of the cable will now be equal to ______.
By increasing the temperature, the specific resistance of a conductor and a semiconductor -
The higher and lower fixed points on a thermometer are separated by 160 mm. When the length of the mercury thread above the lower point is 40 mm, the temperature reading would be :
Water at 10°C enters into a geyser. The water drawn out from the geyser has a temperature of 60°C and the rate of outflow of water is 18 kg/hr. The rating of the geyser is :
The specific resistance of all the metals is the most affected by ______
Temperature dependence of resistivity ρ(T) of semiconductors, insulators and metals is significantly based on the following factors:
- number of charge carriers can change with temperature T.
- time interval between two successive collisions can depend on T.
- length of material can be a function of T.
- mass of carriers is a function of T.
The temperature (T) dependence of resistivity of materials A and material B is represented by fig (i) and fig (ii) respectively. Identify material A and material B.
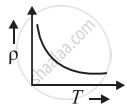 fig. (i) |
 fig. (ii) |
