Advertisements
Advertisements
Question
Find the value of a if the distance between the points (5 , a) and (1 , 5) is 5 units .
Solution
Let the points (5 ,a) and (1 , -5) be P and Q respectively.
Given , PQ = 5 units
`sqrt ((5 - 1)^2 + ("a" + 5)^2) = 5`
squaring both sides , we get ,
16 + a2 + 25 + 10"a" = 25
⇒ a2 + 10a + 16 = 0
⇒ a2 + 8a + 2a + 16 = 0
⇒ (a + 8)(a + 2) = 0
∴ a = - 8 , - 2
APPEARS IN
RELATED QUESTIONS
Find the distance between the following pair of points:
(-6, 7) and (-1, -5)
If the point A(x,2) is equidistant form the points B(8,-2) and C(2,-2) , find the value of x. Also, find the value of x . Also, find the length of AB.
Find the distance of the following point from the origin :
(13 , 0)
A(-2, -3), B(-1, 0) and C(7, -6) are the vertices of a triangle. Find the circumcentre and the circumradius of the triangle.
Prove that the points (1 ,1),(-4 , 4) and (4 , 6) are the certices of an isosceles triangle.
Prove taht the points (-2 , 1) , (-1 , 4) and (0 , 3) are the vertices of a right - angled triangle.
PQR is an isosceles triangle . If two of its vertices are P (2 , 0) and Q (2 , 5) , find the coordinates of R if the length of each of the two equal sides is 3.
The point which lies on the perpendicular bisector of the line segment joining the points A(–2, –5) and B(2, 5) is ______.
Case Study -2
A hockey field is the playing surface for the game of hockey. Historically, the game was played on natural turf (grass) but nowadays it is predominantly played on an artificial turf.
It is rectangular in shape - 100 yards by 60 yards. Goals consist of two upright posts placed equidistant from the centre of the backline, joined at the top by a horizontal crossbar. The inner edges of the posts must be 3.66 metres (4 yards) apart, and the lower edge of the crossbar must be 2.14 metres (7 feet) above the ground.
Each team plays with 11 players on the field during the game including the goalie. Positions you might play include -
- Forward: As shown by players A, B, C and D.
- Midfielders: As shown by players E, F and G.
- Fullbacks: As shown by players H, I and J.
- Goalie: As shown by player K.
Using the picture of a hockey field below, answer the questions that follow:
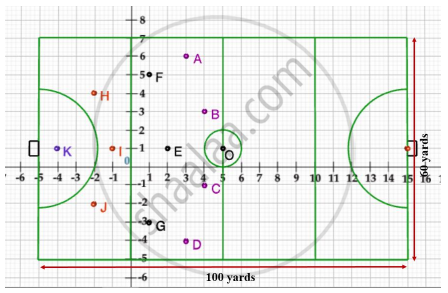
The point on y axis equidistant from B and C is ______.
|
Tharunya was thrilled to know that the football tournament is fixed with a monthly timeframe from 20th July to 20th August 2023 and for the first time in the FIFA Women’s World Cup’s history, two nations host in 10 venues. Her father felt that the game can be better understood if the position of players is represented as points on a coordinate plane. |
- At an instance, the midfielders and forward formed a parallelogram. Find the position of the central midfielder (D) if the position of other players who formed the parallelogram are :- A(1, 2), B(4, 3) and C(6, 6)
- Check if the Goal keeper G(–3, 5), Sweeper H(3, 1) and Wing-back K(0, 3) fall on a same straight line.
[or]
Check if the Full-back J(5, –3) and centre-back I(–4, 6) are equidistant from forward C(0, 1) and if C is the mid-point of IJ. - If Defensive midfielder A(1, 4), Attacking midfielder B(2, –3) and Striker E(a, b) lie on the same straight line and B is equidistant from A and E, find the position of E.

