Advertisements
Advertisements
Question
Find the zeroes of the quadratic polynomial `f(x) = x^2 + 3x ˗ 10` and verify the relation between its zeroes and coefficients.
Solution
We have:
`f(x) = x^2 + 3x ˗ 10`
=` x^2 + 5x ˗ 2x ˗ 10`
= x(x + 5) ˗ 2(x + 5)
= (x ˗ 2) (x + 5)
∴ f(x) = 0 ⇒ (x ˗ 2) (x + 5) = 0
⇒ x ˗ 2 = 0 or x + 5 = 0
⇒ x = 2 or x = −5.
So, the zeroes of f(x) are 2 and −5.
Sum of zeroes= `2+(-5)=-3=(-3)/1="(-coefficient of x)" /(("Coefficent of "x^2))`
Product of zeroes =`2xx(-5)=-10=(-10)/1= "Constant term"/(("Coefficcient of "x^2 ))`
APPEARS IN
RELATED QUESTIONS
The graphs of y = p(x) are given in following figure, for some polynomials p(x). Find the number of zeroes of p(x).
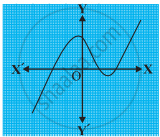
If one zero of the polynomial `x^2-4x+1 is (2+sqrt3)` , write the other zero.
If 1is a zero of the quadratic polynomial `ax^2 – 3(a – 1)x – 1`is 1, then find the value of a.
Write the zeros of the polynomial `f(x) = x^2 – x – 6`.
If the sum of the zeros of the quadratic polynomial `kx^2-3x + 5` is 1 write the value of k..
If 𝛼 and 𝛽 be the zeroes of the polynomial `2x^2 - 7x + k` write the value of (𝛼 + 𝛽+ 𝛼 𝛽.
If ∝ and β are the zeros of the polynomial f(x) = `6x^2 + x - 2 `find the value of `(∝/β+∝/β) `
The number of polynomials having zeroes as -2 and 5 is ______.
A polynomial of degree n has ______.
If the zeroes of the quadratic polynomial ax2 + bx + c, c ≠ 0 are equal, then ______.
