Advertisements
Advertisements
Question
From the curve y = |x|, draw y = |x − 1| + 1
Solution
y = |x|
y = `{{:(x, "if" x ≥ 0),(- x, "if" x < 0):}`
| x | 0 | 1 | 2 | 3 | − 1 | − 2 | − 3 |
| y | 0 | 1 | 2 | 3 | 1 | 2 | 3 |

(a) Consider y = |x – 1|
y = `{{:((x - 1), "if" x - 1 ≥ 0),(-(x - 1), "if" x - 1 < 0):}`
y = `{{:(x - 1, "if" x ≥ 1),(-x + 1, "if" x < 1):}`
x = 0 ⇒ y = – x + 1 ⇒ y = 1
x = 1 ⇒ y = x – 1 ⇒ y = 0
x = 2 ⇒ y = x – 1 ⇒ y = 1
x = 3 ⇒ y = x – 1 ⇒ y = 2
x = – 1 ⇒ y = – x + 1 ⇒ y = 2
x = – 2 ⇒ y = – x + 1 ⇒ y = 3
| x | 0 | 1 | 2 | 3 | − 1 | − 2 |
| y | 1 | 0 | 1 | 2 | 2 | 3 |
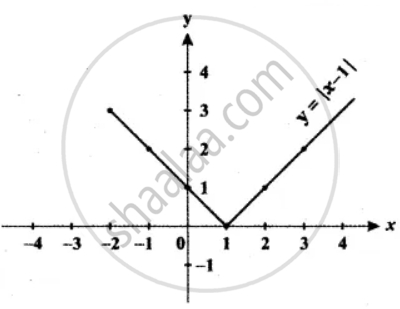
The graph of y = |x – 1| causes the graph y = |x| a shift to the right by 1 unit.
The graph of y = f(x – c), c > 0 causes the graph y = f(x) a shift to the right by c units.
(b) Consider y = |x – 1| + 1
y = `{{:((x - 1) + 1, "if" x - 1 ≥ 0),(-(x - 1) + 1, "if" x - 1 < 0):}`
y = `{{:(x, "if" x ≥ 1),(- x + 2, "if" x < 1):}`
x = 0 ⇒ y = – x + 2 ⇒ y = 2
x = 1 ⇒ y = x ⇒ y = 1
x = 2 ⇒ y = x ⇒ y = 2
x = 3 ⇒ y = x ⇒ y = 3
x = – 1 ⇒ y = – x + 2 ⇒ y = 3
x = – 2 ⇒ y = – x + 2 ⇒ y = 4
| x | 0 | 1 | 2 | 3 | − 1 | − 2 |
| y | 2 | 1 | 2 | 3 | 3 | 4 |

The graph of y = |x – 1| causes the graph y = |x| a shift to the right by 1 unit.
The graph of y = f(x – c), c > 0 causes the graph y = f(x) a shift to the right by c units.
The graph of y = f(x) + d, d > 0 causes the graph y = f(x) a shift to the upward by d units.
APPEARS IN
RELATED QUESTIONS
For the curve y = x3 given in Figure 1.67, draw
y = x3 + 1 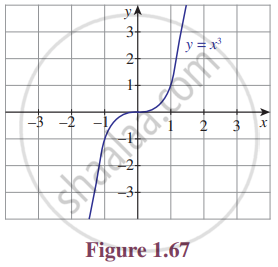
For the curve y = x3 given in Figure 1.67, draw
y = x3 − 1 
For the curve y = `x^((1/3))` given in Figure 1.68, draw
y = `x^((1/3)) + 1`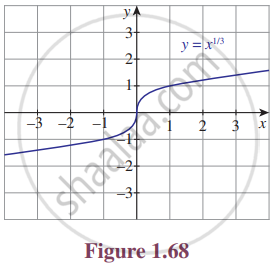
For the curve y = `x^((1/3))` given in Figure 1.68, draw
y = `(x + 1)^((1/3))`
Graph the functions f(x) = x3 and g(x) = `root(3)(x)` on the same coordinate plane. Find f o g and graph it on the plane as well. Explain your results
Write the steps to obtain the graph of the function y = 3(x − 1)2 + 5 from the graph y = x2
From the curve y = sin x, graph the function.
y = sin(− x)
From the curve y = sin x, graph the function
y = `sin(pi/2 + x)` which is cos x
From the curve y = sin x, graph the function
y = `sin (pi/2 - x)` which is also cos x (refer trigonometry)
From the curve y = x, draw y = − x
From the curve y = x, draw y = x + 1
From the curve y = x, draw y = `1/2 x + 1`
From the curve y = x, draw 2x + y + 3 = 0
From the curve y = |x|, draw y = |x + 2| − 3
From the curve y = sin x, draw y = sin |x| (Hint: sin(−x) = − sin x)
