Advertisements
Advertisements
Question
Given that x ∈ I, solve the inequation and graph the solution on the number line: `3 ≥ (x - 4)/(2) + x/(3) ≥ 2`
Solution
`3 ≥ (x - 4)/(2) + x/(3) and 3 ≥ (x - 4)/(2) + x/(3) ≥ 2`
(i) `3 ≥ (3x - 12 + 2x)/(6)`
⇒ `3 ≥ (5x - 12)/(6)`
⇒ 18 ≥ 5x - 12
⇒ 5x - 12 ≤ 18
⇒ 5x ≤ 18 + 12
⇒ 5x ≤ 30
⇒ x ≤ 6
(ii) `(x - 4)/(2) + x/(3) ≥ 2`
`(3x - 12 + 2x)/(6) ≥ 2`
⇒ `(5x - 12)/(6) ≥ 2`
⇒ 5x - 12 ≥ 12
⇒ 5x ≥ 12 + 12, x ≥ `(24)/(5)`
⇒ x ≥ `4(4)/(5)`
∴ x = {5, 6}
Number line: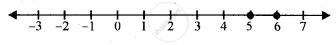
APPEARS IN
RELATED QUESTIONS
Solve the following inequation and write the solution set:
13x – 5 < 15x + 4 < 7x + 12, x ∈ R
Represent the solution on a real number line.
Solve the following in equation and represent the solution set on the number line.
`R - 3 < -1/2 - (2x)/3 <= 5/6, x ∈ R`
For the following inequations, graph the solution set on the real number line:
– 4 ≤ 3x – 1 < 8
Solve the following inequation and write down the solution set:
11x - a <15 x + 4 ≤ 12xk + 14 , x ∈ W
Represent the solution on a real number line.
Graph the solution set for each inequality:
5 ≤ x < 10
Solve the equation and represent the solution set on the number line.
`-3 + x ≤ (8x)/(3)+ 2 ≤ (14)/(3)+ 2x`, where x ∈ I
Solve the following inequalities and represent the solution on a number line:
2x + 3 < 5
Solve 2 ≤ 2x – 3 ≤ 5, x ∈ R and mark it on number line.
Solve the following inequation and represent the solution set on the number line : `4x - 19 < (3x)/(5) - 2 ≤ -(2)/(5) + x, x ∈ "R"`
Solve the following inequation, write down the solution set and represent it on the real number line.
`-3 + x ≤ (7x)/2 + 2 < 8 + 2x, x ∈ I`
