Advertisements
Advertisements
Question
If A(5,2), B(2, -2) and C(-2, t) are the vertices of a right triangle with ∠B=90° , then find the value of t .
Solution
∵ ∠B = 90°
`∴ AC^2 = AB^2+BC^2`
`⇒ (5+2)^2 +(2-t)^2 = (5-2)^2 +(2+2)^2 +(2+2)^2+(-2-t)^2`
`⇒(7)^2 +(t-2)^2 = (3)^2 +(4)^2 +(4)^2 + (t+2)^2`
`⇒ 49+t^2-4t+4=9+16+16+t^2+4t+4`
⇒ 8-4t=4t
⇒ 8t=8
⇒ t=1
Hence, t =1.
APPEARS IN
RELATED QUESTIONS
Prove that the points (2,3), (-4, -6) and (1, 3/2) do not form a triangle.
If `a≠ b ≠ c`, prove that the points (a, a2), (b, b2), (c, c2) can never be collinear.
Show that the points are the vertices of an isosceles right triangle.
Show that the points (-3, -3),(3,3) and C (-3 `sqrt(3) , 3 sqrt(3))` are the vertices of an equilateral triangle.
A(7, -3), B(5,3) and C(3,-1) are the vertices of a ΔABC and AD is its median. Prove that the median AD divides ΔABC into two triangles of equal areas.
Find the value of x for which the points (x, −1), (2, 1) and (4, 5) are collinear ?
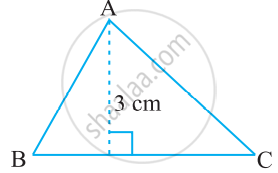
Find BC, if the area of the triangle ABC is 36 cm2 and the height AD is 3 cm.
The table given below contains some measures of the right angled triangle. Find the unknown values.
| Base | Height | Area |
| 5 feet | ? | 20 sq.feet |
In the given figure, if PR = 12 cm, QR = 6 cm and PL = 8 cm, then QM is ______.

Area of a right-angled triangle is 30 cm2. If its smallest side is 5 cm, then its hypotenuse is ______.
