Advertisements
Advertisements
Question
If the sum of the zeros of the quadratic polynomial `kx^2-3x + 5` is 1 write the value of k..
Solution
By using the relationship between the zeroes of the quadratic polynomial.
We have
Product of zeroes=`("Constant term")/("Coefficient of "x^2)`
`⇒ =k/1`
`⇒ k=3`
APPEARS IN
RELATED QUESTIONS
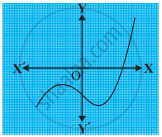
The graphs of y = p(x) are given in following figure, for some polynomials p(x). Find the number of zeroes of p(x).
Find the zeroes of the quadratic polynomial `f(x) = x^2 + 3x ˗ 10` and verify the relation between its zeroes and coefficients.
Find the zeroes of the quadratic polynomial `f(x) = 5x^2 ˗ 4 ˗ 8x` and verify the relationship between the zeroes and coefficients of the given polynomial.
If f(x) =`x^3-3x+5x-3` is divided by g(x)=`x^2-2`
Find all the zeroes of `(2x^4 – 3x^3 – 5x2 + 9x – 3)`, it is being given that two of its zeroes are `sqrt3 and –sqrt3`.
If 3 is a zero of the polynomial `2x^2 + x + k`, find the value of k.
The number of polynomials having zeroes as -2 and 5 is ______.
If 4x² – 6x – m is divisible by x – 3, the value of m is exact divisor of ______.
If α and β are the zeroes of the polynomial x2 – 1, then the value of (α + β) is ______.
The given linear polynomial y = f(x) has