Advertisements
Advertisements
Question
If the length of the diagonal of a cube is `5sqrt(3)` cm, find the total surface area.
Length of the diagonal of the cube = `square`
So, `square` = `5sqrt(3)`
⇒ Side = `square`
Total surface area of cube = `square`
= `square` × `square` × `square`
= `square` cm2
Hence, the total surface area is `square`.
Solution
Length of the diagonal of the cube = `bb(5sqrt(3) cm)`
So, `bb("Side" xx sqrt(3))` = `5sqrt(3)`
⇒ Side = 5
Total surface area of cube = 6 × Side2
= 6 × 5 × 5
= 150 cm2
Hence, the total surface area is 150 m2.
APPEARS IN
RELATED QUESTIONS
Sushant has a vessel, of the form of an inverted cone, open at the top, of height 11 cm and radius of top as 2.5 cm and is full of water. Metallic spherical balls each of diameter 0.5 cm are put in the vessel due to which 2/5 th of the water in the vessel flows out. Find how many balls were put in the vessel. Sushant made the arrangement so that the water that flows out irrigates the flower beds. What value has been shown by Sushant?
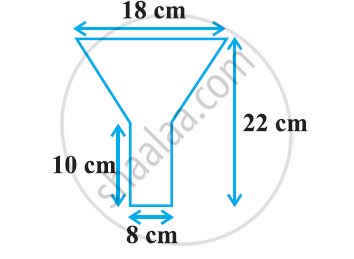
An oil funnel made of tin sheet consists of a 10 cm long cylindrical portion attached to a frustum of a cone. If the total height is 22 cm, diameter of the cylindrical portion is 8 cm and the diameter of the top of the funnel is 18 cm, find the area of the tin sheet required to make the funnel (see the given figure).
Find the volume of a cone if the radius of its base is 1.5 cm and its perpendicular height is 5 cm.
A cylindrical tank full of water is emptied by a pipe at the rate of 225 litres per minute. How much time will it take to empty half the tank, if the diameter of its base is 3 m and its height is 3.5 m? [Use \[\pi = \frac{22}{7}\]]
A spherical shell of lead, whose external diameter is 18 cm, is melted and recast into a right circular cylinder, whose height is 8 cm and diameter 12 cm. Determine the internal diameter of the shell.
Two cubes have their volumes in the ratio 1 : 27. What is the ratio of their surface areas?
A sphere of maximum volume is cut-out from a solid hemisphere of radius r, what is the ratio of the volume of the hemisphere to that of the cut-out sphere?
A solid metallic spherical ball of diameter 6 cm is melted and recast into a cone with diameter of the base as 12 cm. The height of the cone is
A circus tent is cylindrical to a height of 3 m and conical above it. If its base radius is 52.5 m and the slant height of the conical portion is 53 m, find the area of canvas needed to make the tent.
A spherical glass vessel has a cylindrical neck that is 7 cm long and 4 cm in diameter. The diameter of the spherical part is 21 cm. Find the quantity of water the vessel can hold.
A sphere of diameter 18 cm is dropped into a cylindrical vessel of diameter 36 cm, partly filled with water. If the sphere is completely submerged, then the water level rises by ______.
Find the number of solid spheres, each of diameter 6 cm, that could be moulded to form a solid metallic cylinder of height 45 cm and diameter 4 cm.
The surface areas of a sphere and a cube are equal. Find the ratio of their volumes.
A solid is composed of a cylinder with hemispherical ends. If the whole length of the solid is 104 cm and the radius of each hemispherical end is 7 cm, find the surface area of the solid.
Tick the object which has more volume
A metal cuboid of measures 16 cm × 11 cm × 10 cm was melted to make coins. How many coins were made, if the thickness and diameter of each coin was 2 mm and 2 cm respectively? (π = 3.14)
The surface areas of two spheres are in the ratio 1 : 2. The ratio of their volume is ______.
Volumes of two spheres are in the ratio 64 : 27. The ratio of their surface areas is ______.
The surface area of a sphere is 616 sq cm. Find its radius tan β = `3/4`
