Advertisements
Advertisements
Question
In a ∆ABC, AD is the bisector of ∠BAC. If AB = 8 cm, BD = 6 cm and DC = 3 cm. The length of the side AC is
Options
6 cm
4 cm
3 cm
8 cm
Solution
4 cm
Explanation;
Hint: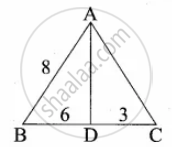
Since AD is the bisector of ∠A
`"BD"/"DC" = "AB"/"AC"`
`6/3 = 8/"AC"`
AC = `(3 xx 8)/6`
= 4 cm
APPEARS IN
RELATED QUESTIONS
In ∆PQR, PQ = √8 , QR = √5 , PR = √3. Is ∆PQR a right-angled triangle? If yes, which angle is of 90°?
In the adjacent figure, ABC is a right angled triangle with right angle at B and points D, E trisect BC. Prove that 8AE2 = 3AC2 + 5AD2

8, 15, 17 is a Pythagorean triplet
Check whether given sides are the sides of right-angled triangles, using Pythagoras theorem
9, 40, 41
Check whether given sides are the sides of right-angled triangles, using Pythagoras theorem
24, 45, 51
Choose the correct alternative:
In right angled triangle, if sum of the squares of the sides of right angle is 169, then what is the length of the hypotenuse?
Choose the correct alternative:
If length of both diagonals of rhombus are 60 and 80, then what is the length of side?
A rectangle having dimensions 35 m × 12 m, then what is the length of its diagonal?
In ∆LMN, l = 5, m = 13, n = 12 then complete the activity to show that whether the given triangle is right angled triangle or not.
*(l, m, n are opposite sides of ∠L, ∠M, ∠N respectively)
Activity: In ∆LMN, l = 5, m = 13, n = `square`
∴ l2 = `square`, m2 = 169, n2 = 144.
∴ l2 + n2 = 25 + 144 = `square`
∴ `square` + l2 = m2
∴By Converse of Pythagoras theorem, ∆LMN is right angled triangle.
In the given figure, triangle PQR is right-angled at Q. S is the mid-point of side QR. Prove that QR2 = 4(PS2 – PQ2).

