Question

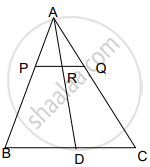
In ΔABC, P and Q are points on AB and AC, respectively, such that PQ is parallel to BC. Prove that the median AD drawn from A on BC bisects PQ.

Answer 1

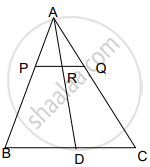
Suppose the median AD intersects PQ at R.

Given,

PQ || BC

or ∠APR = ∠B and ∠AQR = ∠C  ...(Corresponding angles)

So in ΔAPR and ΔABD

∠APR = ∠ABD

∠PAR = ∠BAD

∴ ΔAPR ∼ ΔABD

or, `(PR)/(BD)` = `(AR)/(AD)`  ....(i)

Similarly, ΔAQR ∼ ΔACD

or, `(QR)/(CD)` = `(AR)/(AD)`  ....(ii)

From equation (i) and (ii),

`(PR)/(BD)` = `(QR)/(CD)`

or `(PR)/(BD)` = `(QR)/(CD)`,  as CD = BD

or PR = QR

Hence, AD bisects PQ.

Hence proved.