Advertisements
Advertisements
Question
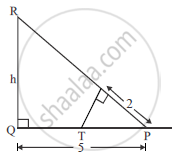
In the given figure, ∠PQR = ∠PST = 90° ,PQ = 5cm and PS = 2cm.
(i) Prove that ΔPQR ~ ΔPST.
(ii) Find Area of ΔPQR : Area of quadrilateral SRQT.

Solution
To prove ΔPQR ∼ ΔPST
In ΔPQR and ΔPST
∠PQR = ∠PST = 90°
∠P = ∠P ( common )
∴ ΔPQR ∼ ΔPST {by AA axiom}
(ii) `("Area of PQR ")/("Area of Quadrilateral")` =
`("ar" (ΔPQR))/("ar" (ΔPST )) = (1/2 xx PQ xx QR )/(1/2 xxPS xx ST) = 5/2 xx 5/2`
`("ar" (ΔPQR))/("ar" (ΔPST )) = 25/4 [ ∵ (PQ)/(PS) = (QR)/(ST) ]`
Taking the reciprocals on both sides
`("ar" (ΔPST ))/("ar" (ΔPQR)) = 4/25`
Now deducting both sides by 1
` therefore 1 - ("ar" (ΔPST ))/("ar" (ΔPQR)) = 1-4/25`
`("ar" (ΔPQR )- "ar" (ΔPST)) /("ar"(ΔPQR)) = 21/25`
`⇒ ("Area of quadrilateral")/("ar"(Delta PQR)) = 21/25`
APPEARS IN
RELATED QUESTIONS
In figure, QA and PB are perpendicular to AB. If AO = 10 cm, BO = 6 cm and PB = 9 cm. Find AQ
The perimeter of two similar triangles are 30 cm and 24 cm. If one side of the first triangle is 12 cm, determine the corresponding side of the second triangle.
The given diagram shows two isosceles triangles which are similar. In the given diagram, PQ and BC are not parallel; PC = 4, AQ = 3, QB = 12, BC = 15 and AP = PQ.

Calculate:
- the length of AP,
- the ratio of the areas of triangle APQ and triangle ABC.
In the given figure, ∠ABC = 90° and BD⊥AC. If AB = 5.7cm, BD = 3.8cm and CD = 5.4cm, find BC.
The areas of two similar triangles are 169cm2 and 121cm2 respectively. If the longest side of the larger triangle is 26cm, find the longest side of the smaller triangle.

O is any point in the interior of ΔABC. Bisectors of ∠AOB, ∠BOC and ∠AOC intersect side AB, side BC, side AC in
F, D and E respectively.
Prove that
BF × AE × CD = AF × CE × BD
In Δ ABC , MN || BC .

If `"AB"/"AM" = 9/4` , find `("Ar" ("trapezium MBCN"))/("Ar" . (triangle "ABC"))`
Let ∆ ABC ∽ ∆ DEF and their areas be respectively, 64 cm2 and 121 cm2. If EF = 15⋅4 cm, find BC.
ABCD is a parallelogram whose sides AB and BC are 18cm and 12cm respectively. G is a point on AC such that CG : GA = 3 : 5 BG is produced to meet CD at Q and AD produced at P. Prove that ΔCGB ∼ ΔAGP. Hence, fi AP.
Prove that if a line is drawn parallel to one side of a triangle intersecting the other two sides in distinct points, then the other two sides are divided in the same ratio.
Using the above theorem prove that a line through the point of intersection of the diagonals and parallel to the base of the trapezium divides the non-parallel sides in the same ratio.
