Advertisements
Advertisements
Question
In a parallelogram ABCD, ∠D = 135°, determine the measure of ∠A and ∠B.
Solution
\[\text{ In a parallelogram, opposite angles have the same value }. \]
\[ \therefore \angle D = \angle B\]
\[ = 135°\]
\[\text{ Also }, \angle A + \angle B + \angle C + \angle D = 360°\]
\[\angle A + \angle D = 180° \left( \text{ Popposite angles have the same value }\right)\]
\[\angle A = 180°- 135° = 45°\]
\[\angle A = 45°\]
APPEARS IN
RELATED QUESTIONS
Which of the following statement is true for a rhombus?
It has two pairs of parallel sides.
Which of the following statement is true for a rhombus?
Two of its angles are at right angles
Which of the following statement is true for a rhombus?
It is a quadrilateral.
If the diagonals of a rhombus are 12 cm and 16cm, find the length of each side.
The diagonals of a quadrilateral are of lengths 6 cm and 8 cm. If the diagonals bisect each other at right angles, what is the length of each side of the quadrilateral?
If all pairs of adjacent sides of a quadrilateral are congruent then it is called ______.
Draw a rhombus KLMN such that its side is 4 cm and m∠K = 75°.
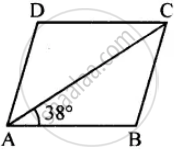
ABCD is a rhombus. If ∠BAC = 38°, find :
(i) ∠ACB
(ii) ∠DAC
(iii) ∠ADC.
If a diagonal of a quadrilateral bisects both the angles, then it is a ______.
All rhombuses are squares.
