Advertisements
Advertisements
Question
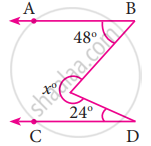
In the figure, AB is parallel to CD, find x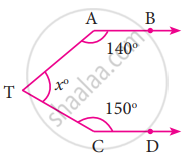
Solution
Through T draw TE || AB.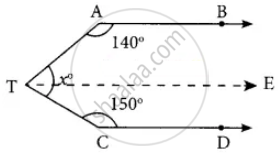
∴ ∠BAT + ∠ATE = 180° ...(AB || TE)
140° + ∠ATE = 180°
∠ATE = 180° − 140° = 40°
Similarly ∠ETC + ∠TCD = 180° ...(TE || CD)
∠ETC + 150° = 180°
∠ETC = 180° − 150° = 30°
x = ∠ATE + ∠ETC
= 40° + 30° = 70°
x = 70°
APPEARS IN
RELATED QUESTIONS
Find the unknown angles in the given figure:
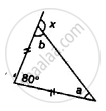
Apply the properties of isosceles and equilateral triangles to find the unknown angles in the given figure:

Find the unknown angles in the given figure:
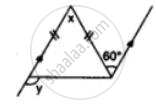
Apply the properties of isosceles and equilateral triangles to find the unknown angles in the given figure:
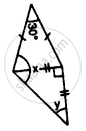
The vertical angle of an isosceles triangle is three times the sum of its base angles. Find each angle.
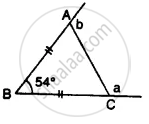
In ∆ ABC, BA and BC are produced. Find the angles a and h. if AB = BC.
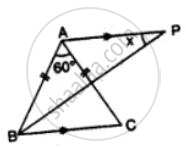
In Figure, BP bisects ∠ABC and AB = AC. Find x.
In the figure, given below, ABCD is a square, and ∆ BEC is an equilateral triangle. Find, the case:∠ABE

In the figure, given below, ABCD is a square, and ∆ BEC is an equilateral triangle. Find, the case:∠BAE
In the figure, AB is parallel to CD, find x