Advertisements
Advertisements
Question
In the figure, AB is parallel to CD, find x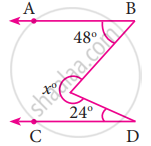
Solution
Draw TE || AB.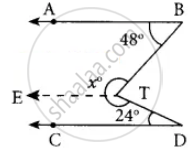
∠ABT + ∠ETB = 180° ...(AB || TE)
48° + ∠ETB = 180°
∠ETB = 180° – 48° = 132°
Similarly ∠CDT + ∠DTE = 180°
24° + ∠DTE = 180°
∴ ∠DTE = 180° – 24°
= 156°
∴ ∠BTE + ∠ETD = 132° + 156°
= 288°
x = 288°
APPEARS IN
RELATED QUESTIONS
Find the unknown angles in the given figure:

Find the unknown angles in the given figure:
Find the unknown angles in the given figure:
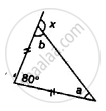
Find the unknown angles in the given figure:

Find the unknown angles in the given figure:
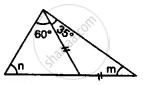
Apply the properties of isosceles and equilateral triangles to find the unknown angles in the given figure:

Apply the properties of isosceles and equilateral triangles to find the unknown angles in the given figure:
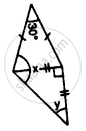
In an isosceles triangle, each base angle is four times its vertical angle. Find all the angles of the triangle.
In the given figure, BI is the bisector of ∠ABC and Cl is the bisector of ∠ACB. Find ∠BIC.
In Figure, BP bisects ∠ABC and AB = AC. Find x.