Advertisements
Advertisements
Question
In the given figure, BI is the bisector of ∠ABC and Cl is the bisector of ∠ACB. Find ∠BIC.
Solution
In Δ ABC,
BI is the bisector of ∠ABC and CI is the bisector of ∠ACB.
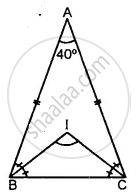
∵ AB = AC
∴ ∠B = ∠C ............(Angles opposite to equal sides)
But ∠A = 40°
and ∠A + ∠B + ∠C = 180° ......(Angles of a triangle)
⇒ 40° + ∠B + ∠B = 180°
⇒ 40° + 2 ∠B = 180°
⇒ 2 ∠B = 180° − 40° = 140°
⇒ ∠B =`(140°)/2=70°`
∴ ∠ABC = ∠ACB = 70°
But BI and CI are the bisectors of ∠ABC and ∠ACB respectively.
∴ ∠IBC =`1/2` ∠ABC =`1/2(70°)=35°`
and ∠ICB =`1/2` ∠ACB =`1/2xx70°=35°`
Now in Δ IBC,
∠BIC + ∠IBC + ∠ICB = 180° ........(Angles of a triangle)
⇒ ∠BIC + 35° + 35° = 180°
⇒ ∠BIC + 70° = 180°
⇒ ∠BIC = 180° − 70° = 110°
Hence ∠BIC = 110°
APPEARS IN
RELATED QUESTIONS
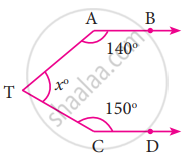
Find the unknown angles in the given figure:
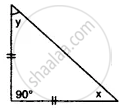
Find the unknown angles in the given figure:
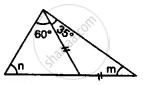
Apply the properties of isosceles and equilateral triangles to find the unknown angles in the given figure:
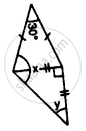
The base angle of an isosceles triangle is 15° more than its vertical angle. Find its each angle.
The vertical angle of an isosceles triangle is three times the sum of its base angles. Find each angle.
In ∆ ABC, BA and BC are produced. Find the angles a and h. if AB = BC.
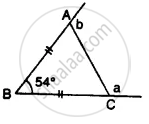
In Figure, BP bisects ∠ABC and AB = AC. Find x.
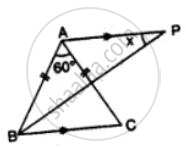
Find x in Figure Given: DA = DB = DC, BD bisects ∠ABC and∠ADB = 70°.
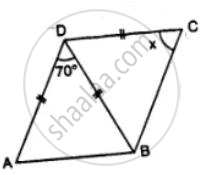
In the figure, given below, ABCD is a square, and ∆ BEC is an equilateral triangle. Find, the case:∠ABE

In the figure, AB is parallel to CD, find x