Advertisements
Advertisements
Question
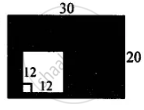
In the figure given below, find the area of shaded region: (All measurements are in cm)
Solution
Outer length = 30 cm
and Outer breadth = 20 cm
∴ Outer area = l × b
= 30 × 20 = 600 cm2
Inner length = 12 cm and inner breadth = 12 cm
Inner area = l × b = 12 × 12 = 144 cm2
Area of shaded portion = Area of outer figure − Area of inner figure
= 600 − 144 = 456 cm2
APPEARS IN
RELATED QUESTIONS
Find the area of the square whose perimeter is 56 cm.
Find the area of the rhombus, if its diagonals are 30 cm and 24 cm.
If the area of a rhombus is 112 cm2 and one of its diagonals is 14 cm, find its other diagonal.
The area of a rhombus is 84 cm2 and its perimeter is 56 cm. Find its height.
Find the area of an equilateral triangle whose each side is 16 cm. (Take `sqrt3`= 1.73)
The legs of a right-angled triangle are in the ratio 4 : 3 and its area is 4056 cm2. Find the length of its legs.
The ratio between the radius of two circles is 5 : 7. Find the ratio between their:
(i) circumference
(ii) areas
A wire is along the boundary of a circle with a radius of 28 cm. If the same wire is bent in the form of a square, find the area of the square formed.
The length and breadth of a rectangular paper are 35 cm and 22 cm. Find the area of the largest circle which can be cut out of this paper.
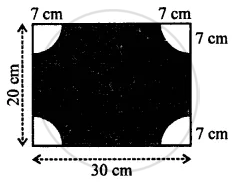
From each corner of a rectangular paper (30 cm x 20 cm) a quadrant of a circle of radius 7 cm is cut. Find the area of the remaining paper i.e., shaded portion.