Advertisements
Advertisements
Question
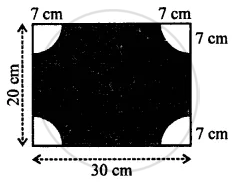
From each corner of a rectangular paper (30 cm x 20 cm) a quadrant of a circle of radius 7 cm is cut. Find the area of the remaining paper i.e., shaded portion.
Solution
Length of paper (l) = 30 cm
and breadth (b) = 20 cm
∴ Area of rectangular paper = l × b
= 30 × 20 = 600 cm
Radius of each quadrant at the corner = 7 cm
Area of 4 quadrants =`4xx1/4π"r"^2`
= πr2 = `22/7xx7xx7` = 154 cm2
∴ Area of remaining paper
= 600 − 154 = 446 cm2
APPEARS IN
RELATED QUESTIONS
Find the area of the square whose perimeter is 56 cm.
A square lawn is surrounded by a path 2.5 m wide. If the area of the path is 165 m2 find the area of the lawn.
In the figure given below, find the area of shaded region: (All measurements are in cm)
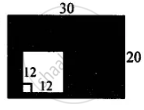
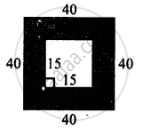
In the figure given below, find the area of shaded region: (All measurements are in cm)
One side of a parallelogram is 18 cm and its area is 153 cm2. Find the distance of the given side from its opposite side.
Find the height of a triangle whose base is 18 cm and the area is 270 cm2.
The area of a right-angled triangle is 160 cm2. If its one leg is 16 cm long, find the length of the other leg.
The area of an equilateral triangle is (`64xxsqrt3`) cm2. Find the length of each side of the triangle.
The ratio between the areas of two circles is 16 : 9. Find the ratio between their :
(i) radius
(ii) diameters
(iii) circumference
A metal wire, when bent in the form of a square of largest area, encloses an area of 484 cm2. Find the length of the wire. If the same wire is bent to the largest circle, find:
(i) radius of the circle formed.
(ii) area of the circle.
