Advertisements
Advertisements
Question
In the given figure, AB || EF || DC; AB = 67.5 cm, DC = 40.5 cm and AE = 52.5 cm.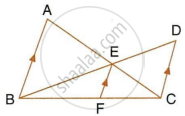
- Name the three pairs of similar triangles.
- Find the lengths of EC and EF.
Solution
i. The three pair of similar triangles are:
∆BEF and ∆BDC
∆CEF and ∆CAB
∆ABE and ∆CDE
ii. Since, ∆ABE and ∆CDE are similar,
`(AB)/(CD) = (AE)/(CE)`
`(67.5)/(40.5) = (52.5)/(CE)`
CE = 31.5 cm
Since, ∆CEF and ∆CAB are similar,
`(CE)/(CA) = (EF)/(AB)`
`(31.5)/(52.5 + 31.5) = (EF)/67.5`
`(31.5)/84 = (EF)/67.5`
`EF = 2126.25/84`
`EF = 405/16 = 25 5/16 cm`
APPEARS IN
RELATED QUESTIONS
The given diagram shows two isosceles triangles which are similar. In the given diagram, PQ and BC are not parallel; PC = 4, AQ = 3, QB = 12, BC = 15 and AP = PQ.

Calculate:
- the length of AP,
- the ratio of the areas of triangle APQ and triangle ABC.
ΔABC~ΔPQR and ar(ΔABC) = 4, ar(ΔPQR) . If BC = 12cm, find QR.
If ΔABC ~ ΔPQR and ∠A = 60°, then ∠P = ?
Points A(3, 1), B(5, 1), C(a, b) and D(4, 3) are vertices of a parallelogram ABCD. Find the values of a and b.
In the adjoining figure. BC is parallel to DE, area of ΔABC = 25 sq cm, area of trapezium BCED = 24 sq cm, DE = 14 cm. Calculate the length of BC.
In ΔABC, point D divides AB in the ratio 5:7, Find: `"AD"/"AB"`
AM and DN are the altitudes of two similar triangles ABC and DEF. Prove that: AM : DN = AB : DE.
ΔABC is enlarged, with a scale factor 5. Find: A'B', if AB = 4cm
If figure OPRQ is a square and ∠MLN = 90°. Prove that QR2 = MQ × RN

In the adjoining diagram the length of PR is ______.

