Advertisements
Advertisements
Question
In the given figure, AC is the diameter of the circle with centre O. If ∠ADE = 30°, ∠DAC = 35° and ∠CAB = 40°. Find ∠ACD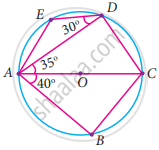
Sum
Solution
∠ADC = 90° ...(Angle in a semi-circle)
∠ADC + ∠ACD + ∠DAC = 180° ...(Sum of the angles of a triangle is 180°)
90° + ∠ACD + 35° = 180°
∠ACD = 180° – 125°
∠ACD = 55°
shaalaa.com
Exterior Angle of a Cyclic Quadrilateral
Is there an error in this question or solution?
APPEARS IN
RELATED QUESTIONS
In the given figure, AC is the diameter of the circle with centre O. If ∠ADE = 30°, ∠DAC = 35° and ∠CAB = 40°. Find ∠ACB
In the given figure, AC is the diameter of the circle with centre O. If ∠ADE = 30°, ∠DAC = 35° and ∠CAB = 40°. Find ∠DAE
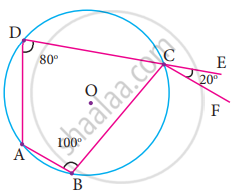
In the figure, ABCD is a cyclic quadrilateral in which DC produced to E and CF is drawn parallel to AB such that ∠ADC = 80° and ∠ECF = 20°, then BAD =?