Advertisements
Advertisements
Question
In the given figure, m(arc WY) = 44°, m(arc ZX) = 68°, then
(1) Find the measure of ∠ ZTX.
(2) If WT = 4.8, TX = 8.0,
YT = 6.4, find TZ.
(3) If WX = 25, YT = 8,
YZ = 26, find WT.

Solution
XW and YZ are two chords of a circle intersecting each other in the interior of the circle at T.
(1)
If two chords of a circle intersect each other in the interior of a circle then the measure of the angle between them is half the sum of measures of arcs intercepted by the angle and its opposite angle.
∴ ∠ZTX = `1/2`[m(arc ZX) + m(arc WY)] =`1/2 xx(68° + 44°) = 1/2 xx 112° = 56°`
Thus, the measure of ∠ZTX is 56º.
(2)
WT × TX = YT × TZ (Theorem of internal division of chords)
⇒ 4.8 × 8 = 6.4 × TZ
⇒ TZ =`(4.8xx8)/6.4 = 6`
(3)
WT × TX = YT × TZ (Theorem of internal division of chords)
⇒ WT × (WX − WT) = YT × (YZ − YT)
⇒ WT × (25 − WT) = 8 × (26 − 8)
⇒ 25WT − WT2 = 8 × 18 = 144
⇒ WT2 − 25WT + 144 = 0
⇒ WT2 − 16WT − 9WT + 144 = 0
⇒ WT(WT − 16) − 9(WT − 16) = 0
⇒ (WT − 16)(WT − 9) = 0
⇒ WT − 16 = 0 or WT − 9 = 0
⇒ WT = 16 or WT = 9
APPEARS IN
RELATED QUESTIONS
Write the length of largest chord of a circle with radius 3.2 cm.
In the above figure, m(arc DXE) = 105°, m(Arc AYC) = 47°, then find the measure of ∠DBE.

Chords AB and CD of a circle intersect inside the circle at point E. If AE = 4, EB = 10, CE = 8, then find ED.
Four alternative answers for the following question are given. Choose the correct alternative and write its alphabet:
A circle having radius 3 cm, then the length of its largest chord is ______.
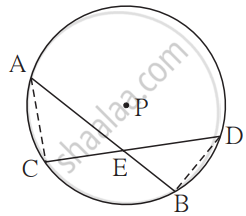
Given:
Chords AB and CD of a circle with centre P intersect at point E.
To prove:
AE × EB = CE × ED
Construction:
Draw seg AC and seg BD.
Fill in the blanks and complete the proof.
Proof:
In Δ CAE and Δ BDE,
∠AEC ≅ ∠DEB ...`square`
`square` ≅ ∠BDE ...(angles inscribed in the same arc)
∴ ΔCAE ~ ΔBDE ...`square`
∴ `square/ ("DE") = ("CE")/square` ...`square`
∴ AE × EB = CE × ED.
