Advertisements
Advertisements
Question
In the given figure, the area of the shaded portion is 770 cm2. If the circumference of the outer circle is 132 cm, find the width of the shaded portion.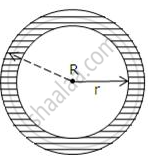
Solution
From the given data, we can calculate the area of the outer circle and then the area of the inner circle and hence the width of the shaded portion.
Given that the circumference of the outer circle is 132 cm
Thus, we have, 2πR = 132 cm
⇒ R = `[ 132 xx 7 ]/[ 2 xx 22 ]`
⇒ R = 21 cm
Area of the bigger circle = πR2
= `22/7` × 212
= 1386 cm2
Also given the area of the shaded portion.
Thus, the area of the inner circle = Area of the outer circle - Area of the shaded portion
= 1386 - 770
= 616 cm2
⇒ πr2 = 616
⇒ r2 = `[ 616 xx 7 ]/22`
⇒ r2 = 196
⇒ r = 14 cm
Thus, the width of the shaded portion = 21 - 14 = 7 cm.
APPEARS IN
RELATED QUESTIONS
A pendulum swings through an angle of 30º and describes an arc 8.8 cm in length. Find the length of the pendulum.
The minute hand of clock is10cm long. Find the area of the face of the clock described by the minute hand between 8am and 8:25 am
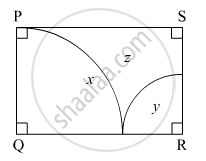
In the given figure,
\[\square\] PQRS is a rectangle. If PQ = 14 cm, QR = 21 cm, find the areas of the parts x, y, and z.
Choose the correct alternative answer for the following question.
A copper wire when bent in the form of a square encloses an area of 484 cm2. The same wire is not bent in the form of a circle. Find the area enclosed by the circle.
The side of a square is 10 cm. Find the area of the circumscribed circle. [π = 3.14]
The sum of diameter of two circles is 112 cm and the sum of their areas is 5236 cm2 Find the radii of the two circles.
Complete the table below.
| Radius (r) | Diameter (d) | Circumference (c) |
| ...... | ...... | 616 cm |
The wheel of a car makes 10 revolutions per second. If its diameter is 70cm, find the speed of the car in km per hr.
The area of a circular playground is 22176 m2. Find the cost of fencing this ground at the rate of Rs 50 per metre.
