Advertisements
Advertisements
Question
The sum of diameter of two circles is 112 cm and the sum of their areas is 5236 cm2 Find the radii of the two circles.
Solution
Sum of the diameters = 112 cm
Therefore, sum of the radii = `112/2` cm = 56 cm
If r is the radius of one circle, radius of other circle is (56 - r)
Sum of the areas = π r2 + π (56 - r)2
⇒ π r2 + π (56 - r)2 = 5236
⇒ π r2 + π (3136 - 112 r + r2) = 5236
⇒ (r2 + 3136 - 112 r + r2) × `22/7` = 5236
⇒ 2r2 - 112r + 3136 = 1666
⇒ 2r2 - 112r + 3136 - 1666 = 0
⇒ 2r2 - 112r + 1470 = 0
⇒ r2 - 56r + 735 = 0
⇒ (r - 35)(r - 21) = 0
⇒ r = 35 , r = 21
Therefore, radii of the two circles is 35 cm and 21 cm
APPEARS IN
RELATED QUESTIONS
In Fig. 7, are shown two arcs PAQ and PBQ. Arc PAQ is a part of circle with centre O and radius OP while arc PBQ is a semi-circle drawn on PQ ad diameter with centre M. If OP = PQ = 10 cm show that area of shaded region is `25(sqrt3-pi/6)cm^2`.

Find the area of the sector of a circle whose radius is 14 cm and angle of sector is 45º
A pendulum swings through an angle of 30º and describes an arc 8.8 cm in length. Find the length of the pendulum.
The area of circle, inscribed in equilateral triangle is 154 cms2. Find the perimeter of
triangle.
The minute hand of a clock is √21 𝑐𝑚 long. Find area described by the minute hand on the face of clock between 7 am and 7:05 am
In circle of radius 6cm, chord of length 10 cm makes an angle of 110° at the centre of circle find The area of sector
The perimeter of a rhombus is 60 cm. If one of its diagonal us 18 cm long, find
(i) the length of the other diagonal, and
(ii) the area of the rhombus.
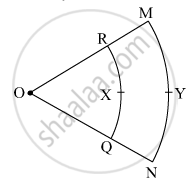
In the given figure, O is the centre of the sector. \[\angle\]ROQ = \[\angle\]MON = 60° . OR = 7 cm, and OM = 21 cm. Find the lengths of arc RXQ and arc MYN. (\[\pi = \frac{22}{7}\])
Find the length of the arc of a circle of diameter 42 cm which subtends an angle of 60° at the centre.
Diameter of the circular garden is 42 m. There is a 3.5 m wide road around the garden. Find the area of the road.

Find the area and perimeter of the circle with the following :
Diameter = 77 cm
Find the area enclosed between two concentric cirdes of radii 6.3 cm and 8.4 cm. A third concentric circle is drawn outside the 8.4 cm circle, so that the area enclosed between it and 8.4 cm cirde is the same as that between two inner circles. Find the radii of the third circle correct to two decimal places .
A cylindrical bucket, whose base radius is 20 cm, is filled with water to a height of 25 cm. A heavy iron spherical ball of radius 10 cm is dropped to submerge completely in water in the bucket. Find the increase in the level of water.
An express train is running between two stations with a uniform speed. If the diameter of each wheel of the train is 42 cm and each wheel makes 1200 revolutions per minute, find the speed of the train.
Find the diameter of a circle whose circumference is equal to the sum of circumference of circles with radius 10 cm, 12 cm, and 18 cm.
Complete the table below.
| Radius (r) | Diameter (d) | Circumference (c) |
| ...... | ...... | 72.6 cm |
Diameters of different circles are given below. Find their circumference (Take π = `22/7`)
d = 28 mm
Find the circumference of the circles whose radii are given below.
49 cm
Circumferences of two circles are equal. Is it necessary that their areas be equal? Why?
A wire is bent to form a square of side 22 cm. If the wire is rebent to form a circle, its radius is ______.
