Advertisements
Advertisements
Question
In the given figure, UB || AT and CU ≡ CB Prove that ΔCUB ~ ΔCAT and hence ΔCAT is isosceles.
Solution
| Statements | Reasons |
| 1. ∠CUB = ∠CBU | ∵ In ΔCUB, CU = CB |
| 2. ∠CUB = ∠CAB | ∵ UB || AT, Corresponding angle if CA is the transversal. |
| 3. ∠CBU = ∠CTA |
CT is transversal UB || AT, Corresponding angle commom angle. |
| 4. ∠UCB = ∠ACT | Common angle |
| 5. ΔCUB ~ ΔCAT | By AAA criteria |
| 6. CA = CT | ∵ ∠CAT = ∠CTA |
| 7. Also ΔCAT is isoceles | By 1, 2 and 3 and sides opposite to equal angles are equal. |
APPEARS IN
RELATED QUESTIONS
The given figure shows a triangle PQR in which XY is parallel to QR. If PX : XQ = 1 : 3 and QR = 9 cm, find the length of XY.

Further, if the area of ΔPXY = x cm2; find, in terms of x the area of :
- triangle PQR.
- trapezium XQRY.
In an isosceles ΔABC, the base AB is produced both ways in P and Q such that
AP × BQ = AC2.
Prove that ΔACP~ΔBCQ.

The scale of a map is 1 : 200000. A plot of land of area 20km2 is to be represented on the map. Find:
The number of kilometres on the ground represented by lcm or the map
In the given figure, PQ || AB; CQ = 4.8 cm QB = 3.6 cm and AB = 6.3 cm. Find :
- `(CP)/(PA)`
- PQ
- If AP = x, then the value of AC in terms of x.

In the figure, PR || SQ. If PR = 10cm, PT = 5cm, TQ = 6cm and ST = 9cm, calculate RT and SQ.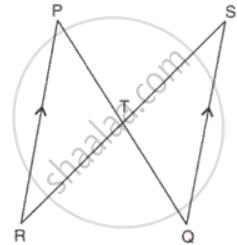
In a quadrilateral PQRS, the diagonals PR and QS intersect each other at the point T. If PT:TR = QT :TS = 1:2, show that TP:TQ = TR:TS
In the figure, which of the following statements is true?
Areas of two similar triangles are equal then prove that triangles are congruent
If ΔABC ∼ ΔDEF and ∠A = 48°, then ∠D = ______.
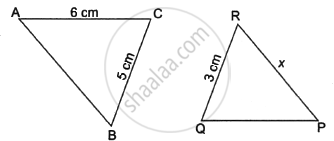
In the given figure, ΔABC ∼ ΔQPR, If AC = 6 cm, BC = 5 cm, QR = 3 cm and PR = x; them the value of x is ______.