Advertisements
Advertisements
Question
Let the period of revolution of a planet at a distance R from a star be T. Prove that if it was at a distance of 2R from the star, its period of revolution will be \[\sqrt{8}\] T.
Solution
From Kepler's third law of planetary motion, we have
or
\[ T_1^2 \propto 8 R^3 . . . . . \](iii)
\[ \Rightarrow T_1 = \sqrt{8}T\]
APPEARS IN
RELATED QUESTIONS
State Kepler's law of orbit and law of equal areas.
A comet orbits the Sun in a highly elliptical orbit. Does the comet have a constant (a) linear speed, (b) angular speed, (c) angular momentum, (d) kinetic energy, (e) potential energy, (f) total energy throughout its orbit? Neglect any mass loss of the comet when it comes very close to the Sun.
State Kepler's laws of planetary motion.
In the Following figure shows the elliptical path of a planet about the sun. The two shaded parts have equal area. If t1 and t2 be the time taken by the planet to go from a to b and from c to d respectively,
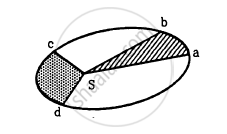
Answer the following question.
State Kepler’s law of equal areas.
Answer the following question.
State Kepler’s law of the period.
Observe the given figure showing the orbit of a planet moving around the Sun and write the three laws related to it:

The orbit of a planet moving around the Sun
If the distance between the sun and the earth is made three times, then attraction between the two will ______
If the sun and the planets carried huge amounts of opposite charges ______.
- all three of Kepler’s laws would still be valid.
- only the third law will be valid.
- the second law will not change.
- the first law will still be valid.
If the sun and the planets carried huge amounts of opposite charges ______.
- all three of Kepler’s laws would still be valid.
- only the third law will be valid.
- the second law will not change.
- the first law will still be valid.
Supposing Newton’s law of gravitation for gravitation forces F1 and F2 between two masses m1 and m2 at positions r1 and r2 read F1 = – F2 = `- r_12/r_12^3 GM_0^2 ((m_1m_2)/M_0^2)^n` where M0 is a constant of dimension of mass r12 = r1 – r2 and n is a number. in such a case.
- the acceleration due to gravity on earth will be different for different objects.
- none of the three laws of Kepler will be valid.
- only the third law will become invalid.
- for n negative, an object lighter than water will sink in water.
The centre of mass of an extended body on the surface of the earth and its centre of gravity ______.
- are always at the same point for any size of the body.
- are always at the same point only for spherical bodies.
- can never be at the same point.
- is close to each other for objects, say of sizes less than 100 m.
- both can change if the object is taken deep inside the earth.
Give one example each of central force and non-central force.
Draw areal velocity versus time graph for mars.
What is the direction of areal velocity of the earth around the sun?
A planet revolving in an elliptical orbit has:
- a constant velocity of revolution.
- has the least velocity when it is nearest to the sun.
- its areal velocity is directly proportional to its velocity.
- areal velocity is inversely proportional to its velocity.
- to follow a trajectory such that the areal velocity is constant.
Choose the correct answer from the options given below:
lf the angular momentum of a planet of mass m, moving around the Sun in a circular orbit is L, about the center of the Sun, and its areal velocity is ______.
Two planets A and B of equal mass are having their period of revolutions TA and TB such that TA = 2TB. These planets are revolving in the circular orbits of radii rA and rB respectively. Which out of the following would be the correct relationship of their orbits?
