Advertisements
Advertisements
Question
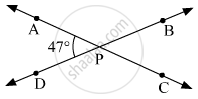
Lines AC and BD intersect at point P. m∠APD = 47°. Find the measures of ∠APB, ∠BPC, ∠CPD.
Solution
In the given figure,
∠DPA + ∠APB = 180∘ (Linear Pair angles)
⇒ 47∘ + ∠APB = 180∘
⇒ ∠APB = 133∘
Now,
∠APD = ∠BPC = 47∘ (Vertically opposite angles)
∠APB = ∠DPC = 133∘ (Vertically opposite angles)
Hence, the measures of ∠APB, ∠BPC, ∠CPD are 133∘, 47∘ and 133∘ respectively.
RELATED QUESTIONS
Find the value of the angles x, y, and z in the following
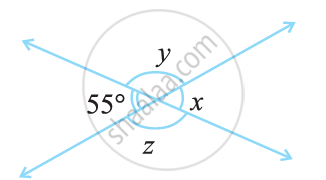
Find the value of the angles x, y, and z in the following:

Fill in the blanks:
If two lines intersect at a point, then the vertically opposite angles are always ______
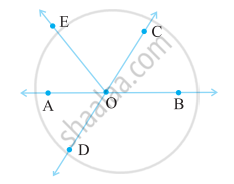
In the given figure, Identify two pairs of vertically opposite angles.
From the given figure, find the missing angle
Using the figure, answer the following questions
(i) What is the measure of angle x°?
(ii) What is the measure of angle y°?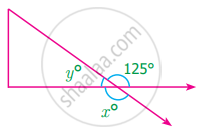
In the given figure, ∠8 = 107°, what is the sum of the ∠2 and ∠4?
Statements a and b are as given below:
a: If two lines intersect, then the vertically opposite angles are equal.
b: If a transversal intersects, two other lines, then the sum of two interior angles on the same side of the transversal is 180°.
Then ______.
Vertically opposite angles are either both acute angles or both obtuse angles.
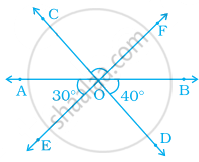
Three lines AB, CD and EF intersect each other at O. If ∠AOE = 30° and ∠DOB = 40° (Figure), find ∠COF.