Advertisements
Advertisements
Question
Measures of opposite angles of a parallelogram are (3x − 2)° and (50 − x)°. Find the measure of its each angle.
Solution
Let ABCD be the parallelogram.
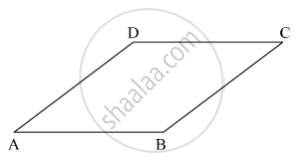
Suppose ∠A = (3x − 2)º and ∠C = (50 − x)º.
We know that the opposite angles of a paralelogram are congruentes.
∴ m∠A = m∠C
⇒ 3x − 2 = 50 − x
⇒ 3x + x = 50 + 2
⇒ 4x = 52
⇒ x = `52/4`
⇒ x = 13
∴ m∠A = (3x − 2)º = (3 × 13 − 2)º = (39 − 2)º = 37º
So, m∠C = m∠A = 37º
Also, the adjacent angles of a parallelogram are supplementary.
∴ m∠A + m∠D = 180º
⇒ 37º + m∠D = 180º
⇒ m∠D = 180º − 37º = 143º
Now,
m∠B = m∠D = 143º (Opposite angles of a parallelogram are congruent)
Thus, the measure of the angles of the parallelogram are 37º, 143º, 37º and 143º.
RELATED QUESTIONS
All rhombuses are kites.
Which of the following figures satisfy the following property?
- Has two pairs of congruent adjacent sides.
Which one has all the properties of a kite and a parallelogram?
A quadrilateral that is not a parallelogram but has exactly two opposite angles of equal measure is ______.
If only one diagonal of a quadrilateral bisects the other, then the quadrilateral is known as ______.
Every kite is a parallelogram.
A playground in the town is in the form of a kite. The perimeter is 106 metres. If one of its sides is 23 metres, what are the lengths of other three sides?
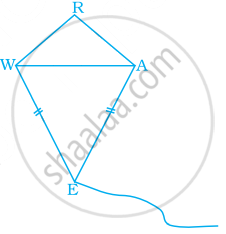
In kite WEAR, ∠WEA = 70° and ∠ARW = 80°. Find the remaining two angles.
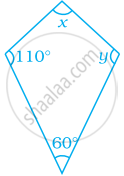
Find the values of x and y in the following kite.
All kites are rhombuses.
