Advertisements
Advertisements
Question
P(1, -2) is a point on the line segment A(3, -6) and B(x, y) such that AP : PB is equal to 2 : 3. Find the coordinates of B.
Solution
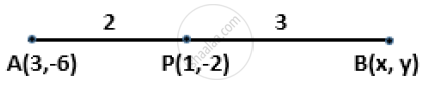
Given, P(1, -2), A(3, -6) and B(x,y)
AP:PB = 2:3
Hence, coordinates of P = `((2xxx+3xx3)/(2+3), (2xxy+3xx(-6))/(2+3)) = ((2x + 9)/5, (2y- 18)/5)`
But, the coordinates of P are (1, 2).
∴ `(2x + 9)/5 = 1` and `(2y - 18)/5 = -2`
⇒ 2x + 9 = 5 and 2y - 18 =-10
⇒ 2x = -4 and 2y = 8
⇒ x = -2 and y = 4
Hence, the coordinates of B are (-2,4).
APPEARS IN
RELATED QUESTIONS
Calculate the co-ordinates of the point P which divides the line segment joining: A (1, 3) and B (5, 9) in the ratio 1 : 2
In what ratio is the line joining (2, –3) and (5, 6) divided by the x-axis?
In what ratio does the point (1, a) divide the join of (–1, 4) and (4, –1)? Also, find the value of a.
If the abscissa of a point P is 2, find the ratio in which this point divides the line segment joining the point (−4, 3) and (6, 3). Also, find the co-ordinates of point P.
M is the mid-point of the line segment joining the points A(0, 4) and B(6, 0). M also divides the line segment OP in the ratio 1 : 3. Find :
- co-ordinates of M
- co-ordinates of P
- length of BP

Prove that the points A(-5, 4), B(-1, -2) and C(S, 2) are the vertices of an isosceles right-angled triangle. Find the coordinates of D so that ABCD is a square.
Show that the points A(1, 3), B(2, 6), C(5, 7) and D(4, 4) are the vertices of a rhombus.
= `a(1/t^2 + 1) = (a(t^2 + 1))/t^2`
Now `(1)/"SP" + (1)/"SQ" = (1)/(a(t^2 + 1)) + (1 xx t^2)/(a(t^2 + 1)`
= `((1 + t^2))/(a(t^2 + 1)`
`(1)/"SP" + (1)/"SQ" = (1)/a`.
The midpoint of the line segment AB shown in the diagram is (4, – 3). Write down the coordinates of A and B.
Determine the centre of the circle on which the points (1, 7), (7 – 1), and (8, 6) lie. What is the radius of the circle?
From the adjacent figure:
(i) Write the coordinates of the points A, B, and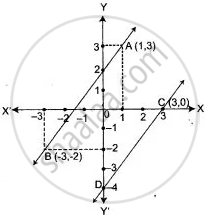
(ii) Write the slope of the line AB.
(iii) Line through C, drawn parallel to AB, intersects Y-axis at D. Calculate the co-ordinates of D.
