Advertisements
Advertisements
Question
Point P(0, 2) is the point of intersection of y-axis and perpendicular bisector of line segment joining the points A(–1, 1) and B(3, 3).
Options
True
False
Solution
This statement is False.
Explanation:
We know that, the points lying on perpendicular bisector of the line segment joining the two points is equidistant from the two points.
i.e., PA should be equals to the PB.
Using distance formula,
d = `sqrt((x_2 - x_1)^2 + (y_2 - y_1)^2`
PA = `sqrt([-4 - (4)]^2 + (6 - 2)^2`
PA = `sqrt((0)^2 + (4)^2` = 4
PB = `sqrt([-4 - 4]^2 + (-6 - 2)^2`
PB = `sqrt(0^2 + (-8)^2` = 8
∵ PA ≠ PB
APPEARS IN
RELATED QUESTIONS
Check whether (5, -2), (6, 4) and (7, -2) are the vertices of an isosceles triangle.
An equilateral triangle has two vertices at the points (3, 4) and (−2, 3), find the coordinates of the third vertex.
Show that the points A(1, 2), B(1, 6), C(1 + 2`sqrt3`, 4) are vertices of an equilateral triangle.
Find the distance between the following point :
(Sin θ - cosec θ , cos θ - cot θ) and (cos θ - cosec θ , -sin θ - cot θ)
Show that the points (2, 0), (–2, 0), and (0, 2) are the vertices of a triangle. Also, a state with the reason for the type of triangle.
The distance between the points (3, 1) and (0, x) is 5. Find x.
Give the relation that must exist between x and y so that (x, y) is equidistant from (6, -1) and (2, 3).
If the distance between the points (4, P) and (1, 0) is 5, then the value of p is ______.
Find a point which is equidistant from the points A(–5, 4) and B(–1, 6)? How many such points are there?
Read the following passage:
|
Alia and Shagun are friends living on the same street in Patel Nagar. Shagun's house is at the intersection of one street with another street on which there is a library. They both study in the same school and that is not far from Shagun's house. Suppose the school is situated at the point O, i.e., the origin, Alia's house is at A. Shagun's house is at B and library is at C. |
Based on the above information, answer the following questions.
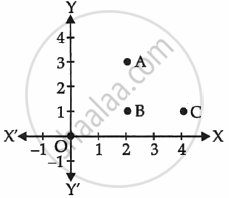
- How far is Alia's house from Shagun's house?
- How far is the library from Shagun's house?
- Show that for Shagun, school is farther compared to Alia's house and library.
OR
Show that Alia’s house, shagun’s house and library for an isosceles right triangle.
