Advertisements
Advertisements
Question
Show that the points (2, 0), (–2, 0), and (0, 2) are the vertices of a triangle. Also, a state with the reason for the type of triangle.
Solution
A ≡ (2, 0) ≡ (x1, y1)
B ≡ (–2 , 0) ≡ (x2, y2)
C ≡ (0, 2) ≡ (x3, y3)
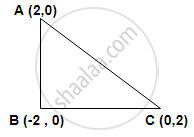
∴ ABC form a triangle.
AB = `sqrt ((x_2 - x_1)^2 + ("y"_2 - "y"_1)^2)`
= `sqrt ((-2-2)^2 + (0 - 0)^2)`
= `sqrt ((-4)^2 + 0)`
= `sqrt(16)`
= 4 units
AC = `sqrt ((x_3 - x_1)^2 + ("y"_3 - "y"_1)^2)`
= `sqrt ((0-2)^2 + (2 - 0)^2)`
= `sqrt ((-2)^2 + (2)^2)`
= `sqrt (4 + 4)`
= `sqrt(8)`
= `2sqrt(2)` units
BC = `sqrt ((x_3 - x_2)^2 + ("y"_3 - "y"_2)^2)`
= `sqrt ((0-(-2)^2) + (2 - 0)^2)`
= `sqrt ((0 + 2)^2 + (2 - 0)^2)`
= `sqrt ((2)^2 + (2)^2)`
= `sqrt(8)`
= `2sqrt(2)` units
So, if side AC and side BC are equal then the triangle is an isosceles triangle.
AB2 = BC2 + AC2
`(4)^2 = (2sqrt2)^2 + (2sqrt(2))^2`
16 = 8 + 8
16 = 16
So, it is a right-angle isosceles triangle.
APPEARS IN
RELATED QUESTIONS
The x-coordinate of a point P is twice its y-coordinate. If P is equidistant from Q(2, –5) and R(–3, 6), find the coordinates of P.
Check whether (5, -2), (6, 4) and (7, -2) are the vertices of an isosceles triangle.
In a classroom, 4 friends are seated at the points A, B, C and D as shown in the following figure. Champa and Chameli walk into the class and after observing for a few minutes, Champa asks Chameli, “Don’t you think ABCD is a square?” Chameli disagrees.
Using distance formula, find which of them is correct.

Name the type of quadrilateral formed, if any, by the following point, and give reasons for your answer:
(4, 5), (7, 6), (4, 3), (1, 2)
Find the point on the x-axis which is equidistant from (2, -5) and (-2, 9).
ABC is a triangle and G(4, 3) is the centroid of the triangle. If A = (1, 3), B = (4, b) and C = (a, 1), find ‘a’ and ‘b’. Find the length of side BC.
An equilateral triangle has two vertices at the points (3, 4) and (−2, 3), find the coordinates of the third vertex.
A(–8, 0), B(0, 16) and C(0, 0) are the vertices of a triangle ABC. Point P lies on AB and Q lies on AC such that AP : PB = 3 : 5 and AQ : QC = 3 : 5. Show that : PQ = `3/8` BC.
If A and B are the points (−6, 7) and (−1, −5) respectively, then the distance
2AB is equal to
If the point P(2, 1) lies on the line segment joining points A(4, 2) and B(8, 4), then ______.
Find the distance of the following point from the origin :
(13 , 0)
Find the distance between the following point :
(Sin θ - cosec θ , cos θ - cot θ) and (cos θ - cosec θ , -sin θ - cot θ)
Find the distance between the following pairs of points:
(-3, 6) and (2, -6)
Find the coordinates of the points on the y-axis, which are at a distance of 10 units from the point (-8, 4).
Calculate the distance between A (7, 3) and B on the x-axis whose abscissa is 11.
Find distance between point A(– 3, 4) and origin O
The distance between the points (0, 5) and (–5, 0) is ______.
Point P(0, 2) is the point of intersection of y-axis and perpendicular bisector of line segment joining the points A(–1, 1) and B(3, 3).
Ayush starts walking from his house to office. Instead of going to the office directly, he goes to a bank first, from there to his daughter’s school and then reaches the office. What is the extra distance travelled by Ayush in reaching his office? (Assume that all distances covered are in straight lines). If the house is situated at (2, 4), bank at (5, 8), school at (13, 14) and office at (13, 26) and coordinates are in km.
A point (x, y) is at a distance of 5 units from the origin. How many such points lie in the third quadrant?
