Advertisements
Advertisements
Question
PQRS is a trapezium having PS and QR as parallel sides. A is any point on PQ and B is a point on SR such that AB || QR. If area of ΔPBQ is 17cm2, find the area of ΔASR.
Advertisements
Solution
Given: Here from the given figure we get
(1) PQRS is a trapezium having PS||QR
(2) A is any point on PQ
(3) B is any point on SR
(4) AB||QR
(5) Area of ΔBPQ = 17 cm2
To find : Area of ΔASR
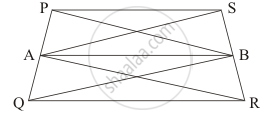
Calculation: We know that ‘If a triangle and a parallelogram are on the same base and the same parallels, the area of the triangle is equal to half the area of the parallelogram’
Here we can see that:
Area (ΔAPB) = Area (ΔABS) …… (1)
And, Area (ΔAQR) = Area (ΔABR) …… (2)
Therefore,
Area (ΔASR) = Area (ΔABS) + Area (ΔABR)
From equation (1) and (2), we have,
Area (ΔASR) = Area (ΔAPB) + Area (ΔAQR)
⇒ Area (ΔASR) = Area (ΔBPQ) = 17 cm2
Hence, the area of the triangle ΔASR is 17 cm2.
APPEARS IN
RELATED QUESTIONS
Compute the area of trapezium PQRS is Fig. below.
ABCD is a parallelogram. E is a point on BA such that BE = 2 EA and F is a point on DC
such that DF = 2 FC. Prove that AE CF is a parallelogram whose area is one third of the
area of parallelogram ABCD.
D is the mid-point of side BC of ΔABC and E is the mid-point of BD. if O is the mid-point
of AE, prove that ar (ΔBOE) = `1/8` ar (Δ ABC).
The figure obtained by joining the mid-points of the adjacent sides of a rectangle of sides 8 cm and 6 cm is ______.
The diagonal of a rectangular board is 1 m and its length is 96 cm. Find the area of the board.
Altogether how many squares can be arranged on it?
Look at the table. If you were to write the area of each of these which column would you choose? Make a (✓).
| Square cm |
Square meter |
Square km |
|
| Handkerchief | ✓ | ||
| Sari | |||
| Page of your book | |||
| School land | |||
| Total land of a city | |||
| Door of your classroom | |||
| Chair seat | |||
| Blackboard | |||
| Indian flag | |||
| Land over which a river flows |
Each line gives a story. You have to choose the question which makes the best story problem. The first one is already marked.
- A shopkeeper has 50 boxes. There are 48 fruits in one box.
Tick the one question which matches with the given problem.
Explain why (a) and (c) are not good choices.a) How much will the shopkeeper pay in all? b) How many fruits are there in all? ✓ c) How many more boxes will he need?
Find all the possible dimensions (in natural numbers) of a rectangle with a perimeter 36 cm and find their areas.
Find the area of the following figure by counting squares:
