Advertisements
Advertisements
Question
Prove that any three points on a circle cannot be collinear.
Solution
Let A, B and C be any three points on a circle. Suppose these three points A, B and C on the circle are collinear.
Therefore, the perpendicular bisectors of the chords AB and BC must be parallel because two or more lines which are perpendicular to a given line are parallel to each other.
Now, AB and BC are the chords of the circle. We know that the perpendicular bisector of the chord of a circle passing through its centre.
So, the perpendicular bisectors of the chords AB and BC must intersect at the centre of the circle.
This is a contradiction to our statement that the perpendicular bisectors of AB and BC must be parallel, as parallel lines do not intersect at a point.
Hence, our assumption that three points A, B and C on the circle are collinear is not correct.
Thus, any three points on a circle cannot be collinear.
APPEARS IN
RELATED QUESTIONS
Suppose you are given a circle. Give a construction to find its centre.
If two circles intersect at two points, prove that their centres lie on the perpendicular bisector of the common chord.
Fill in the blank:
All points lying inside/outside a circle are called .................. points/ .....................points.
true or false
Line segment joining the centre to any point on the circle is a radius of the circle,
Give a method to find the centre of a given circle.
A circular park of radius 40 m is situated in a colony. Three boys Ankur, Amit and Anand are sitting at equal distance on its boundary each having a toy telephone in his hands to talk
to each other.
Choose the correct alternative:
If the points, A, B, C are non-collinear points, then how many circles can be drawn which passes through points A, B, and C?
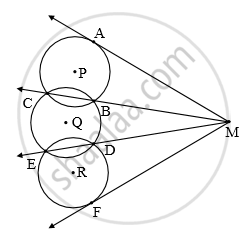
In the above figure, the circles with P, Q, and R intersect at points B, C, D, and E as shown. Lines CB and ED intersect in point M. Lines are drawn from point M to touch the circles at points A and F. Prove that MA = MF.
Four alternative answers for the following question is given. Choose the correct alternative.
How many circles can drawn passing through three non-collinear points?
How many circles can be drawn passing through a point?
Through three collinear points a circle can be drawn.
ABCD is such a quadrilateral that A is the centre of the circle passing through B, C and D. Prove that ∠CBD + ∠CDB = `1/2` ∠BAD
Find the value of y, if the points A(3, 4), B(6, y) and C(7, 8) are collinear.
