Advertisements
Advertisements
Question
ABCD is such a quadrilateral that A is the centre of the circle passing through B, C and D. Prove that ∠CBD + ∠CDB = `1/2` ∠BAD
Advertisements
Solution
In a circle, ABCD is a quadrilateral having center A.
To prove ∠CBD + ∠CDB = `1/2` ∠BAD
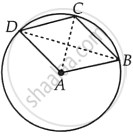
Construction: Join AC.
Proof: As we know that angle subtended by an arc at the center is double the angle subtended by it at point on the remaining part of the circle.
So, ∠CAD = 2∠CBD ...(i)
And ∠BAC = 2∠CDB ...(ii)
Now, adding equation (i) and (ii), we get
∠CAD + ∠BAC = 2(∠CBD + ∠CDB)
∠BAD = 2(∠CBD + ∠CDB)
Hence, ∠CBD + ∠CDB = `1/2` ∠BAD.
APPEARS IN
RELATED QUESTIONS
Draw different pairs of circles. How many points does each pair have in common? What is the maximum number of common points?
Suppose you are given a circle. Give a construction to find its centre.
If two circles intersect at two points, prove that their centres lie on the perpendicular bisector of the common chord.
Fill in the blank:
All points lying inside/outside a circle are called .................. points/ .....................points.
true or false
Line segment joining the centre to any point on the circle is a radius of the circle,
Prove that any three points on a circle cannot be collinear.
Choose the correct alternative:
If the points, A, B, C are non-collinear points, then how many circles can be drawn which passes through points A, B, and C?
In the above figure, the circles with P, Q, and R intersect at points B, C, D, and E as shown. Lines CB and ED intersect in point M. Lines are drawn from point M to touch the circles at points A and F. Prove that MA = MF.
Four alternative answers for the following question is given. Choose the correct alternative.
How many circles can drawn passing through three non-collinear points?
Find the value of y, if the points A(3, 4), B(6, y) and C(7, 8) are collinear.
