Advertisements
Advertisements
प्रश्न
ABCD is such a quadrilateral that A is the centre of the circle passing through B, C and D. Prove that ∠CBD + ∠CDB = `1/2` ∠BAD
उत्तर
In a circle, ABCD is a quadrilateral having center A.
To prove ∠CBD + ∠CDB = `1/2` ∠BAD
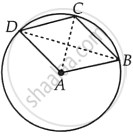
Construction: Join AC.
Proof: As we know that angle subtended by an arc at the center is double the angle subtended by it at point on the remaining part of the circle.
So, ∠CAD = 2∠CBD ...(i)
And ∠BAC = 2∠CDB ...(ii)
Now, adding equation (i) and (ii), we get
∠CAD + ∠BAC = 2(∠CBD + ∠CDB)
∠BAD = 2(∠CBD + ∠CDB)
Hence, ∠CBD + ∠CDB = `1/2` ∠BAD.
APPEARS IN
संबंधित प्रश्न
Draw different pairs of circles. How many points does each pair have in common? What is the maximum number of common points?
Suppose you are given a circle. Give a construction to find its centre.
Fill in the blank:
All points lying inside/outside a circle are called .................. points/ .....................points.
Give a method to find the centre of a given circle.
A circular park of radius 40 m is situated in a colony. Three boys Ankur, Amit and Anand are sitting at equal distance on its boundary each having a toy telephone in his hands to talk
to each other.
Prove that any three points on a circle cannot be collinear.
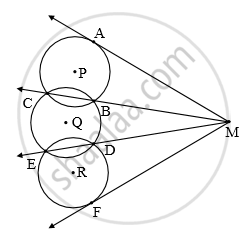
In the above figure, the circles with P, Q, and R intersect at points B, C, D, and E as shown. Lines CB and ED intersect in point M. Lines are drawn from point M to touch the circles at points A and F. Prove that MA = MF.
Four alternative answers for the following question is given. Choose the correct alternative.
How many circles can drawn passing through three non-collinear points?
Through three collinear points a circle can be drawn.
Find the value of y, if the points A(3, 4), B(6, y) and C(7, 8) are collinear.
