Advertisements
Advertisements
प्रश्न
If a line segment joining mid-points of two chords of a circle passes through the centre of the circle, prove that the two chords are parallel.
Advertisements
उत्तर
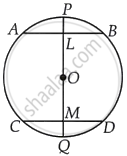
Given: AB and CD are two chords of a circle whose centre is O and PQ is a diameter bisecting the chord AB and CD at L and M, respectively and the diameter PQ passes through the centre O of the circle.
To prove: AB || CD
Proof: Since, L is the mid-point of AB.
∴ OL ⊥ AB ...[Since, the line joining the centre of a circle to the mid-point of a chord is perpendicular to the chord]
⇒ ∠ALO = 90° ...(i)
Similarly, OM ⊥ CD
∴ ∠OMD = 90° ...(ii)
From equations (i) and (ii),
∠ALO = ∠OMD = 90°
But, these are alternating angles.
So, AB || CD
Hence proved.
APPEARS IN
संबंधित प्रश्न
Prove that the intercept of a tangent between two parallel tangents to a circle subtends a right angle at center.
PQ is a chord of length 4.8 cm of a circle of radius 3 cm. The tangents at P and Q intersect at a point T as shown in the figure. Find the length of TP.
In the given figure, a circle inscribed in a triangle ABC touches the sides AB, BC and CA at points D, E and F respectively. If AB = 14 cm, BC = 8 cm and CA = 12 cm. Find the lengths AD, BE and CF.
PQ is a chord of length 8 cm of a circle of radius 5 cm. The tangents at P and Q intersect at a point T. Find the lengths of TP and TQ.
Equal circles with centres O and O' touch each other at X. OO' produced to meet a circle with centre O', at A. AC is a tangent to the circle whose centre is O. O'D is perpendicular to AC. Find the value of\[\frac{DO'}{CO}\]
Find the area of the shaded region in the figure If ABCD is a rectangle with sides 8 cm and 6 cm and O is the centre of the circle. (Take π= 3.14)
State, if the following statement is true or false:
If the end points A and B of the line segment lie on the circumference of a circle, AB is a diameter.
The radius of a circle of diameter 24 cm is _______
Find the radius of the circle
Diameter = 30 cm
If a number of circles pass through the endpoints P and Q of a line segment PQ, then their centres lie on the perpendicular bisector of PQ.
