Advertisements
Advertisements
प्रश्न
In the given figure, O is the centre of the circle. Find ∠CBD.
Advertisements
उत्तर
It is given that, `angle AOC = 100°`
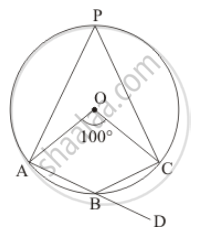
We have to find `angleCBD`
Since `angleAOC = 100°` (Given)
So,
`angleAPC = 1/2 angleAOC` (The angle subtended by an arc of a circle at the centre is double the angle subtended by it at any point on the remaining part of the circle.)
`⇒ angleAPC = 1/2 xx 100`
= 50°
Now,
`angle APC + angleABC = 180° ` (Opposite pair of angle of cyclic quadrilateral)
So,
`50°+ angleABC = 180° `
`angleABC = 180° - 50°`
= 130°
⇒`angle ABC` = 130° …… (1)
`angle ABC + angleCBD = 180° ` (Linear pair)
`130° + angle CBD = 180° ( angleABC = 130)`
`angle CBD = 180° - 130° `
= 50°
Hence `angle CBD ` = 50°
APPEARS IN
संबंधित प्रश्न
PA and PB are tangents from P to the circle with centre O. At point M, a tangent is drawn cutting PA at K and PB at N. Prove that KN = AK + BN.
Fill in the blanks:
The centre of a circle lies in ____________ of the circle.
The lengths of three consecutive sides of a quadrilateral circumscribing a circle are 4 cm, 5 cm and 7 cm respectively. Determine the length of the fourth side.
In the adjoining figure, a circle touches all the four sides of a quadrilateral ABCD whose sides are AB = 6 cm, BC = 9 cm and CD = 8 cm. Find the length of side AD.
Two concentric circles are of diameters 30 cm and 18 cm. Find the length of the chord of the larger circle which touches the smaller circle.
In the given figure, OQ : PQ = 3.4 and perimeter of Δ POQ = 60 cm. Determine PQ, QR and OP.
In the given figure, BC is a tangent to the circle with centre O. OE bisects AP. Prove that ΔAEO ∼ Δ ABC.
ABC is a right triangle in which ∠B = 90°. If AB = 8 cm and BC = 6 cm, find the diameter of the circle inscribed in the triangle.
Draw a circle of diameter 7 cm. Draw two radii of this circle such that the angle between these radii is 90°. Shade the minor sector obtained. Write a special name for this sector.
In the following figure, ∠OAB = 30º and ∠OCB = 57º. Find ∠BOC and ∠AOC.
