Advertisements
Advertisements
Question
|
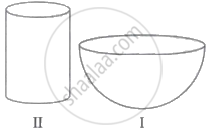
Khurja is a city in the Indian state of Uttar Pradesh famous for the pottery. Khurja pottery is traditional Indian pottery work which has attracted Indians as well as foreigners with a variety of tea sets, crockery and ceramic tile works. A huge portion of the ceramics used in the country is supplied by Khurja and is also referred as "The Ceramic Town". One of the private schools of Bulandshahr organised an Educational Tour for class 10 students to Khurja. Students were very excited about the trip. Following are the few pottery objects of Khurja.
Students found the shapes of the objects very interesting and they could easily relate them with mathematical shapes viz sphere, hemisphere, cylinder etc. |
Maths teacher who was accompanying the students asked the following questions:
- The internal radius of hemispherical bowl (filled completely with water) in I is 9 cm and the radius and height of the cylindrical jar in II are 1.5 cm and 4 cm respectively. If the hemispherical bowl is to be emptied in cylindrical jars, then how many cylindrical jars are required?
- If in the cylindrical jar full of water, a conical funnel of the same height and same diameter is immersed, then how much water will flow out of the jar?
Solution
a. Given, the radius by the hemispherical bowl, r1 = 9 cm
Radius of the cylindrical jar, r2 = 1.5 cm
Height of cylindrical jar, h2 = 4 cm
Now, Volume of hemispherical bowl = `2/3 πr_1^3`
= `2/3 π(9)^3`
And Volume of cylindrical jar = `πr_2^2h_2`
= π(1.5)2 × 4
Required number of cylindrical jar = `"Volume of hemispherical bowl"/"Volume of cylindrical jar"`
= `(2/3 π(9)^3)/(π(1.5)^2 xx 4)`
= `(2 xx 9 xx 9 xx 9)/(3 xx 1.5 xx 1.5 xx 4)`
= `(3 xx 9 xx 9 xx 10 xx 10)/(15 xx 15 xx 2)`
= `(24,300)/450`
= 54
Hence, 54 cylindrical jars are required.
b. Volume of water flow out of the jar = Volume of the conical funnel
= `1/3 πr_2^2h_2`
= `1/3 xx22/7 xx (1.5)^2 xx 4`
= `1/3 xx 22/7 xx 1.5 xx 1.5 xx 4`
= `(22 xx 15 xx 15 xx 4)/(3 xx 7 xx 10 xx 10)`
= `19800/2100`
= 9.43 cubic cm
Therefore, the water flowing out of the jar is 9.43 cubic cm.
APPEARS IN
RELATED QUESTIONS
The sum of the radius of base and height of a solid right circular cylinder is 37 cm. If the total surface area of the solid cylinder is 1628 sq. cm, find the volume of the cylinder. `("use " pi=22/7)`
The diameters of the lower and upper ends of a bucket in the form of a frustum of a cone are 10 cm and 30 cm respectively. If its height is 24 cm, find:
1) The area of the metal sheet used to make the bucket.
2) Why we should avoid the bucket made by ordinary plastic? [Use π = 3.14]
The internal and external diameters of a hollow hemisphere vessel are 21cm and 25.2 cm The cost of painting 1cm2 of the surface is 10paise. Find total cost to paint the vessel all
over______?
A solid is in the form of a right circular cylinder, with a hemisphere at one end and a cone at the other end. The radius of the common base is 3.5 cm and the heights of the cylindrical and conical portions are 10 cm. and 6 cm, respectively. Find the total surface area of the solid. (Use π =`22/7`)
Two cubes each of volume 27 cm3 are joined end to end to form a solid. Find the surface area of the resulting cuboid.
How many spherical lead shots each of diameter 4.2 cm can be obtained from a solid rectangular lead piece with dimension 6cm \[\times\] 42cm \[\times\] 21 cm.
A solid cuboid of iron with dimensions 53 cm ⨯ 40 cm ⨯ 15 cm is melted and recast into a cylindrical pipe. The outer and inner diameters of pipe are 8 cm and 7 cm respectively. Find the length of pipe.
A solid metallic sphere of diameter 28 cm is melted and recast into a number of smaller cones, each of diameter 4 \[\frac{2}{3}\] cm and height 3 cm. Find the number of cones so formed.
Match the following columns:
| Column I | Column II |
| (a) The radii of the circular ends of a bucket, in the form of the frustum of a cone of height 30 cm, are 20 cm and 10 cm respectively. The capacity of the bucket is ........cm3. |
(p) 2418π |
| (b) The radii of the circular ends of a conical bucket of height 15 cm are 20 and 12 cm respectively. The slant height of the bucket is ........ cm. |
(q) 22000 |
| (c) The radii of the circular ends of a solid frustum of a cone are 33 cm and 27 cm and its slant height is 10 cm. The total surface area of the bucket is .........cm2. |
(r) 12 |
| (d) Three solid metallic spheres of radii 3 cm, 4 cm and 5 cm are melted to form a single solid sphere. The diameter of the resulting sphere is ........ cm. |
(s) 17 |
Two cubes each of volume 8 cm³ are joined end to end, then the surface area of the resulting cuboid is ______.