Advertisements
Advertisements
प्रश्न
|
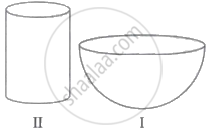
Khurja is a city in the Indian state of Uttar Pradesh famous for the pottery. Khurja pottery is traditional Indian pottery work which has attracted Indians as well as foreigners with a variety of tea sets, crockery and ceramic tile works. A huge portion of the ceramics used in the country is supplied by Khurja and is also referred as "The Ceramic Town". One of the private schools of Bulandshahr organised an Educational Tour for class 10 students to Khurja. Students were very excited about the trip. Following are the few pottery objects of Khurja.
Students found the shapes of the objects very interesting and they could easily relate them with mathematical shapes viz sphere, hemisphere, cylinder etc. |
Maths teacher who was accompanying the students asked the following questions:
- The internal radius of hemispherical bowl (filled completely with water) in I is 9 cm and the radius and height of the cylindrical jar in II are 1.5 cm and 4 cm respectively. If the hemispherical bowl is to be emptied in cylindrical jars, then how many cylindrical jars are required?
- If in the cylindrical jar full of water, a conical funnel of the same height and same diameter is immersed, then how much water will flow out of the jar?
उत्तर
a. Given, the radius by the hemispherical bowl, r1 = 9 cm
Radius of the cylindrical jar, r2 = 1.5 cm
Height of cylindrical jar, h2 = 4 cm
Now, Volume of hemispherical bowl = `2/3 πr_1^3`
= `2/3 π(9)^3`
And Volume of cylindrical jar = `πr_2^2h_2`
= π(1.5)2 × 4
Required number of cylindrical jar = `"Volume of hemispherical bowl"/"Volume of cylindrical jar"`
= `(2/3 π(9)^3)/(π(1.5)^2 xx 4)`
= `(2 xx 9 xx 9 xx 9)/(3 xx 1.5 xx 1.5 xx 4)`
= `(3 xx 9 xx 9 xx 10 xx 10)/(15 xx 15 xx 2)`
= `(24,300)/450`
= 54
Hence, 54 cylindrical jars are required.
b. Volume of water flow out of the jar = Volume of the conical funnel
= `1/3 πr_2^2h_2`
= `1/3 xx22/7 xx (1.5)^2 xx 4`
= `1/3 xx 22/7 xx 1.5 xx 1.5 xx 4`
= `(22 xx 15 xx 15 xx 4)/(3 xx 7 xx 10 xx 10)`
= `19800/2100`
= 9.43 cubic cm
Therefore, the water flowing out of the jar is 9.43 cubic cm.
APPEARS IN
संबंधित प्रश्न
Due to sudden floods, some welfare associations jointly requested the government to get 100 tents fixed immediately and offered to contribute 50% of the cost. If the lower part of each tent is of the form of a cylinder of diameter 4.2 m and height 4 m with the conical upper part of same diameter but of height 2.8 m, and the canvas to be used costs Rs. 100 per sq. m, find the amount, the associations will have to pay. What values are shown by these associations? [Use π=22/7]
A toy is in the form of a cone of base radius 3.5 cm mounted on a hemisphere of base diameter 7 cm. If the total height of the toy is 15.5 cm, find the total surface area of the top (Use π = 22/7)
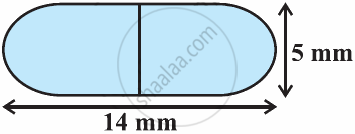
A medicine capsule is in the shape of cylinder with two hemispheres stuck to each of its ends (see the given figure). The length of the entire capsule is 14 mm and the diameter of the capsule is 5 mm. Find its surface area. [Use π = `22/7`]
The perimeters of the ends of a frustum of a right circular cone are 44 cm and 33 cm. If the height of the frustum be 16 cm, find its volume, the slant surface and the total surface.
A cylindrical tub, whose diameter is 12 cm and height 15 cm is full of ice-cream. The whole ice-cream is to be divided into 10 children in equal ice-cream cones, with conical base surmounted by hemispherical top. If the height of conical portion is twice the diameter of base, find the diameter of conical part of ice-cream cone ?
If the total surface area of a solid hemisphere is 462 cm2, then find its volume.
A toy is in the form of a cylinder with hemispherical ends. If the whole length of the toy is 90 cm and its diameter is 42 cm, then find the cost of painting the toy at the rate of 70 paise per sq cm.
A cubical block of side 10 cm is surmounted by a hemisphere. What is the largest diameter that the hemisphere can have? Find the cost of painting the total surface area of the solid so formed, at the rate of ₹5 per 100 sq cm. [Use ππ = 3.14]
Find the ratio of the volume of a cube to that of a sphere which will fit inside it.
A bucket open at the top is in the form of a frustum of a cone with a capacity of 12308.8 cm3. The radii of the top and bottom of the circular ends of the bucket are 20 cm and 12 cm respectively. Find the height of the bucket and also the area of the metal sheet used in making it. (Use π = 3.14)