Advertisements
Advertisements
प्रश्न
Due to sudden floods, some welfare associations jointly requested the government to get 100 tents fixed immediately and offered to contribute 50% of the cost. If the lower part of each tent is of the form of a cylinder of diameter 4.2 m and height 4 m with the conical upper part of same diameter but of height 2.8 m, and the canvas to be used costs Rs. 100 per sq. m, find the amount, the associations will have to pay. What values are shown by these associations? [Use π=22/7]
उत्तर
Diameter of the tent = 4.2 m
Radius of the tent, r = 2.1 m
Height of the cylindrical part of tent, hcylinder = 4 m
Height of the conical part, hcone = 2.8 m
Slant height of the conical part, l
`=sqrt(h_(`
`=sqrt((2.8)^2+(2.1)^2)`
`=sqrt(12.5)=3.5m`
Curved surface area of the cylinder = 2𝜋r hcylinder
= 2 ×(22/7)× 2.1 × 4
= 22 × 0.3 × 8 = 52.8 m2
Curved surface area of the conical tent = 𝜋rl =(22/7)× 2.1 × 3.5 = 23.1 m2
Total area of cloth required for building one tent
= Curved surface area of the cylinder + Curved surface area of the conical tent
= 52.8 + 23.1
= 75.9 m2
Cost of building one tent = 75.9 × 100 = Rs. 7590
Total cost of 100 tents = 7590 × 100 = Rs. 7,59,000
Cost to be borne by the associations =759000/2= Rs. 3,79,500
It shows the helping nature, unity and cooperativeness of the associations.
APPEARS IN
संबंधित प्रश्न
The sum of the radius of base and height of a solid right circular cylinder is 37 cm. If the total surface area of the solid cylinder is 1628 sq. cm, find the volume of the cylinder. `("use " pi=22/7)`
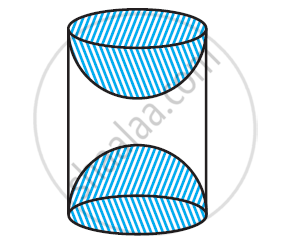
A wooden article was made by scooping out a hemisphere from each end of a solid cylinder, as shown in given figure. If the height of the cylinder is 10 cm, and its base is of radius 3.5 cm, find the total surface area of the article.
[Use `pi = 22/7`]
A bucket made of aluminum sheet is of height 20cm and its upper and lower ends are of radius 25cm an 10cm, find cost of making bucket if the aluminum sheet costs Rs 70 per
100 cm2
If the radius of the base of a right circular cylinder is halved, keeping the height the same, then the ratio of the volume of the cylinder thus obtained to the volume of original cylinder is:
Two cubes each of volume 27 cm3 are joined end to end to form a solid. Find the surface area of the resulting cuboid.
The inner and outer radii of a hollow cylinder are 15 cm and 20 cm, respectively. The cylinder is melted and recast into a solid cylinder of the same height. Find the radius of the base of new cylinder.
A cylindrical bucket 28 cm in diameter and 72 cm high is full of water. The water is emptied into a rectangular tank 66 cm long and 28 cm wide. Find the height of the water level in the tank.
Two cubes each of volume 27 cm3 are joined end to end to form a solid. Find the surface area of the resulting cuboid.
A hemispherical bowl of internal diameter 30 cm contains some liquid. This liquid is to be poured into cylindrical bottles of diameter 5 cm and height 6 cm each. Find the number of bottles required.
If the surface areas of two spheres are in ratio 16 : 9, then their volumes will be in the ratio ______.
