Advertisements
Advertisements
प्रश्न
If the radius of the base of a right circular cylinder is halved, keeping the height the same, then the ratio of the volume of the cylinder thus obtained to the volume of original cylinder is:
विकल्प
1 : 2
2 : 1
1 : 4
4 : 1
उत्तर
Let the radius and height of the original cylinder be r and h respectively.
∴Volume of the original cylinder = πr2h
According to the question, radius of the new cylinder is halved keeping the height same.
⇒ Radius of the new cylinder`=r/2`
Also, height of the new cylinder = h
∴ Volume of the new cylinder `pi(r/2)^2h=(pir^2h)/4`
`\text{Volume of the new cylinder}/\text{Volume of original cylinder}=(((pir^2h)/4))/(r^2h4)=underline1=1:4`
Hence, the correct answer is C.
APPEARS IN
संबंधित प्रश्न
If the total surface area of a solid hemisphere is 462 cm2 , find its volume.[Take π=22/7]
2 cubes each of volume 64 cm3 are joined end to end. Find the surface area of the resulting cuboid.
A toy is in the shape of a right circular cylinder with a hemisphere on one end and a cone on the other. The radius and height of the cylindrical part are 5 cm and 13 cm respectively.The radii of the hemispherical and conical parts are the same as that of the cylindrical part.Find the surface area of the toy if the total height of the toy is 30 cm.
A solid metallic sphere of diameter 28 cm is melted and recast into a number of smaller cones, each of diameter 4 \[\frac{2}{3}\] cm and height 3 cm. Find the number of cones so formed.
A solid is hemispherical at the bottom and conical above. If the surface areas of the two parts are equal, then the ratio of its radius and the height of its conical part is
The volume of a hemisphere is 19404 cm3. The total surface area of the hemisphere is
A cone of height 24 cm and radius of base 6 cm is made up of modelling clay. A child reshapes it in the form of a sphere. Find the radius of the sphere and hence find the surface area of this sphere.
Two cones with same base radius 8 cm and height 15 cm are joined together along their bases. Find the surface area of the shape so formed.
|
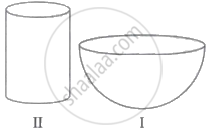
Khurja is a city in the Indian state of Uttar Pradesh famous for the pottery. Khurja pottery is traditional Indian pottery work which has attracted Indians as well as foreigners with a variety of tea sets, crockery and ceramic tile works. A huge portion of the ceramics used in the country is supplied by Khurja and is also referred as "The Ceramic Town". One of the private schools of Bulandshahr organised an Educational Tour for class 10 students to Khurja. Students were very excited about the trip. Following are the few pottery objects of Khurja.
Students found the shapes of the objects very interesting and they could easily relate them with mathematical shapes viz sphere, hemisphere, cylinder etc. |
Maths teacher who was accompanying the students asked the following questions:
- The internal radius of hemispherical bowl (filled completely with water) in I is 9 cm and the radius and height of the cylindrical jar in II are 1.5 cm and 4 cm respectively. If the hemispherical bowl is to be emptied in cylindrical jars, then how many cylindrical jars are required?
- If in the cylindrical jar full of water, a conical funnel of the same height and same diameter is immersed, then how much water will flow out of the jar?
3 cubes each of 8 cm edge are joined end to end. Find the total surface area of the cuboid.