Advertisements
Advertisements
Question
Solve any two of the following.
Solve : `3x+y=14 ; x-y=2`
Solution
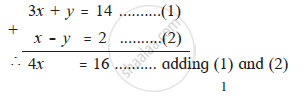
`∴ x=4`
substituting x = 4 in equation (2)
`4-y=2`
`∴-y=2-4=-2`
`∴ y=2`
APPEARS IN
RELATED QUESTIONS
A train covers a distance of 300 km at a uniform speed. If the speed of the train is increased by 5 km/hour, it takes 2 hours less in the journey. Find the original speed of the train
Which of the following is a quadratic equation?
(a)` (x^2+1)=(2-x)^2+3` (b)` x^3-x^2=(x-1)^3`
(c) `2x^+3=(5+x)(2x-3)` (d) None of these
If the product of the roots of the equation `x^2-3x+k=10` is-2 then the value of k is
(a) -2 (b) -8 (c) 8 (d) 12
Solve `x^2-(sqrt3+1)x+sqrt3=0`
Solve for x: `3x^2-2sqrt6x+2=0`
Decide whether the following equation is quadratic equation or not.
m3 + 3m2 – 2 = 3m3
Write the following equations in the form ax2 + bx + c= 0, then write the values of a, b, c for each equation.
p(3+6p) = –5
The Sum of squares of 2 consecutive natural even numbers is 244; find the numbers.
In the orange garden of Mr. Madhusudan there are 150 orange trees. The number of trees in each row is 5 more than that in each column. Find the number of trees in each row and each column with the help of following flow chart.

Vivek is older than Kishor by 5 years. The sum of the reciprocals of their ages is \[\frac{1}{6}\] Find their present ages.
Suyash scored 10 marks more in second test than that in the first. 5 times the score of the second test is the same as square of the score in the first test. Find his score in the first test.
Pintu takes 6 days more than those of Nishu to complete certain work. If they work together they finish it in 4 days. How many days would it take to complete the work if they work alone.
Choose the correct answer for the following question.
Out of the following equations which one is not a quadratic equation?
Choose the correct answer for the following question.
For \[\sqrt{2} x^2 - 5x + \sqrt{2} = 0\] find the value of the discriminant.
A tank fills completely in 2 hours if both the taps are open. If only one of the taps is open at the given time, the smaller tap takes 3 hours more than the larger one to fill the tank. How much time does each tap take to fill the tank completely?
Solve the following quadratic equation.
\[x^2 - \frac{3x}{10} - \frac{1}{10} = 0\]
Alka spends 90 % of the amount sent to her and saves Rs. 120 per month. Find the amount sent to her per month.
Two water taps together can fill a tank in `1(7)/(8)` hours. The tap with longer diameter takes 2 hours less than the tap with a smaller one to fill the tank separately. Find the time in which each tap can fill the tank separately.
Solve for x : `1/(2a + b + 2x) =1/(2a) + 1/b + 1/(2x); x ≠ 0, x ≠ (−2a −b)/2`, a, b ≠ 0
If 460 is divided by a natural number, then quotient is 2 more than nine times the divisor and remainder is 5. Find the quotient and divisor.
