Advertisements
Advertisements
Question
Solve the following inequation and represent the solution set on the number line : `-3 < -(1)/(2) - (2x)/(3) ≤ (5)/(6), x ∈ "R"`
Solution
`-3 < -(1)/(2) - (2x)/(3) ≤ (5)/(6), x ∈ "R"`
(i) `-3 < -(1)/(2) - (2x)/(3)`
⇒ `-3 ≤ - (1/2 + (2x)/3)`
⇒ `- (1/2 + (2x)/3) > -3`
⇒ `-(2x)/(3) > -3 + (1)/(2)`
⇒ `-(2x)/(3) > (-5)/(2)`
⇒ `(2x)/(3) < (5)/(2)`
⇒ `x < (5)/(2) xx (3)/(2)`
⇒ `x < (15)/(4)` ....(i)
(ii) `-(1)/(2) - (2x)/(3) ≤ (5)/(6)`
⇒ `-(2x)/(3) ≤ (5)/(6) + (1)/(2)`
⇒ `(-2x)/(3) ≤ (5 + 3)/(6)`
⇒ `(-2)/(3) xx ≤ (8)/(6)`
⇒ `(2)/(3)x ≥ (-8)/(6)`
⇒ `x ≥ (-8)/(6) xx (3)/(2)`
⇒ x ≥ -2
⇒ -2 ≤ x ....(ii)
⇒ From (i) and (ii),
`-2 ≤ ≤ (15)/(4)`
∴ Solution = `{ x : x ∈ "R", -2 ≤ x < (15)/(4)}`
Now solution on number line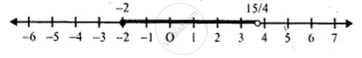
APPEARS IN
RELATED QUESTIONS
Solve the following inequation and write the solution set:
13x – 5 < 15x + 4 < 7x + 12, x ∈ R
Represent the solution on a real number line.
Represent the following inequalities on real number line:
– 4 < x < 4
Represent the following inequalities on real number line:
8 ≥ x > – 3
Use the real number line to find the range of values of x for which:
x > 3 and 0 < x < 6
Solve the following in equation and write the solution set:
13x - 5 < 15x + 4 < 7x + 12, x ∈ R
Represent the solution on a real number line.
Graph the solution set for each inequality:
-3< x <5
Given:
P = {x : 5 < 2x - 1 ≤ 11, x ∈ R}
Q = {x : -1 ≤ 3 + 4x < 23, x ∈ R}
Where R = (real number), I = (Integers) Reperesnr P and Q on number lines. Write down the elements of P ∩ Q.
Solve the following inequalities and represent the solution on a number line:
2x + 3 < 5
The solution set for the following number line is:
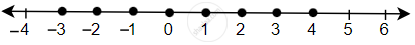
The number line for the solution of inequation x > 5 and x < 10 (x ∈ R) is:
