Advertisements
Advertisements
Question
The area of a square is given by 4x2 + 12xy + 9y2. Find the side of the square.
Solution
We have,
Area of square = 4x2 + 12xy + 9y2
So, we factorise the given expression.
∴ 4x2 + 12xy + 9y2 = (2x)2 + 2 × 2x × 3y + (3y)2 ...[∵ a2 + 2ab + b2 = (a + b)2]
= (2x + 3y)2
Since, area of a square having side length a is a2.
Hence, side of the given square is 2x + 3y.
APPEARS IN
RELATED QUESTIONS
Use a suitable identity to get the following products.
(6x − 7) (6x + 7)
Expand (ax + by)2
Use an expansion formula to find the value.
(97)2
Use the formula to multiply the following.
(x + y) (x − y)
Evaluate the following, using suitable identity
512
Factorise the following using suitable identity
a2 + 6ab + 9b2 – c2
Factorise the following, using the identity a2 + 2ab + b2 = (a + b)2.
x2 + 12x + 36
Factorise the following, using the identity a2 + 2ab + b2 = (a + b)2.
a2x2 + 2ax + 1
What should be added to 4c(– a + b + c) to obtain 3a(a + b + c) – 2b(a – b + c)?
Take suitable number of cards given in the adjoining diagram [G(x × x) representing x2, R(x × 1) representing x and Y(1 × 1) representing 1] to factorise the following expressions, by arranging the cards in the form of rectangles: x2 + 4x + 4. Factorise 2x2 + 6x + 4 by using the figure.
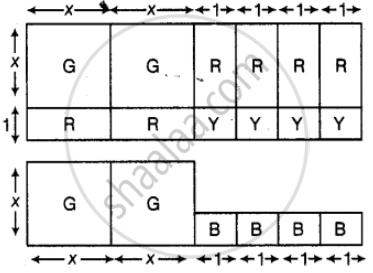
Calculate the area of figure.
