Question

The centre of a circle is (2a, a – 7). Find the values of a if the circle passes through the point (11, – 9) and has diameter `10sqrt(2)` units.

Answer 1

By given condition,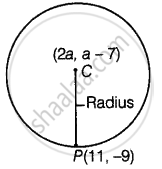

Distance between the centre C(2a, a – 7) and the point P(11, – 9), which lie on the circle = Radius of circle

∴ Radius of circle = `sqrt((11 - 2a)^2 + (-9 - a + 7)^2`   ...(i) `[∵ "Distance between two points"  (x_1, y_1)  "and"  (x_2, y_2) = sqrt((x_2 - x_1)^2 + (y_2 - y_1)^2)]`

Given that, length of diameter = `10sqrt(2)`

∴ Length of radius = `"Length of diameter"/2`

= `(10sqrt(2))/2`

= `5sqrt(2)`

Put this value in equation (i), we get

`5sqrt(2) = sqrt((11 - 2a)^2 + (-2 - a)^2`

Squaring on both sides, we get

50 = (11 – 2a)² + (2 + a)²

⇒ 50 = 121 + 4a² – 44a + 4 + a² + 4a

⇒ 5a² – 40a + 75 = 0

⇒ a² – 8a + 15 = 0

⇒ a² – 5a – 3a + 15 = 0   ...[By fractorisation method]

⇒ a(a – 5) – 3(a – 5) = 0

⇒ (a – 5)(a – 3) = 0

∴ a = 3, 5

Hence, the required values of a are 5 and 3.