Advertisements
Advertisements
Question
The circumference of a circle is 100 m. The side of a square inscribed in the circle is
Options
`50sqrt2`
`50/π`
`(50sqrt(2))/π`
`(100sqrt(2))/π`
Solution
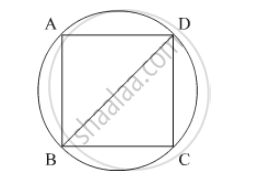
2πr = 100
r = `100/(2π)`
= `50/π`
diagram = `100/π`
`(100/π)^2` = 2 side2
`1000/(π^(2).2)` = side2
side = `sqrt(1000/(π^(2).2))`
`100/π sqrt(1/2)`
= `100/(πsqrt2)`
`(50sqrt2)/π`
APPEARS IN
RELATED QUESTIONS
A circular flower garden has an area of 314 m2. A sprinkler at the centre of the garden can cover an area that has a radius of 12 m. Will the sprinkler water the entire garden? (Take π = 3.14)
In the following figure a square OABC is inscribed in a quadrant OPBQ of a circle. If OA = 21 cm, find the area of the shaded region.

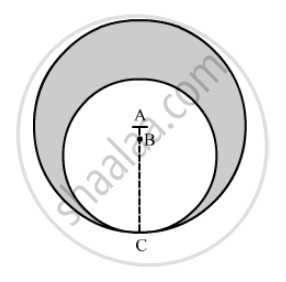
In the following figure, two circles with centres A and B touch each other at the point C. If AC = 8 cm and AB = 3 cm, find the area of the shaded region.
How many revolutions a circular wheel of radius r metres makes in covering a distance of s metres?
The area of the sector of a circle of radius 10.5 cm is 69.3 cm2. Find the central angle of the sector.
A piece of wire of length 108 cm is bent to form a semicircular arc bounded by its diameter. Find its radius and area enclosed.
Find the area enclosed between two concentric circles, If their radii are 6cm and 13cm respectively.
A lawn is in the shape of a semicircle of diameter 42m. the lawn is surrounded by a flower bed of width 7m all round. Find the area of the flower bed in m2.
Find the area of a circle of radius 30 cm (use π = 3.14).
Diameter of a circular garden is 9.8 m. Find its area.
