Advertisements
Advertisements
Question
The co-ordinates of two points A and B are (–3, 4) and (2, –1). Find:
- the equation of AB;
- the co-ordinates of the point where the line AB intersects the y-axis.
Solution
i. Given, co-ordinates of two points A and B are (–3, 4) and (2, –1).
Slope = `(-1 - 4)/(2 + 3) = (-5)/5 = -1`
The equation of the line AB is given by:
y − y1 = m(x − x1)
y + 1 = −1(x − 2)
y + 1 = −x + 2
x + y = 1
ii. Let the line AB intersects the y-axis at point (0, y).
Putting x = 0 in the equation of the line, we get,
0 + y = 1
y = 1
Thus, the co-ordinates of the point where the line AB intersects the y-axis are (0, 1).
APPEARS IN
RELATED QUESTIONS
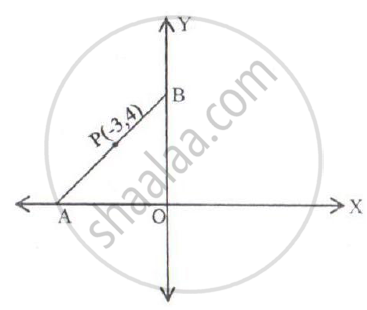
In the figure given below, the line segment AB meets X-axis at A and Y-axis at B. The point P(-3, 4) on AB divides it in the ratio 2:3. Find the coordinates of A and B.
Solve the following inequation and represent the solution set on the number line 2x – 5 <= 5x + 4 < 11, where x ∈ I
State, true or false :
The line `x/2 + y/3 = 0` passes through the point (4, −6).
Find the equation of the line whose slope is `-4/3` and which passes through (−3, 4).
Find the equation of a line which passes through (5, 4) and makes an angle of 60° with the positive direction of the x-axis.
Find the equation of the line, whose x-intercept = 5 and y-intercept = 3
Determine whether the line through points (–2, 3) and (4, 1) is perpendicular to the line 3x = y + 1.
Does line 3x = y + 1 bisect the line segment joining the two given points?
A line intersects x-axis at point (−2, 0) and cuts off an intercept of 3 units from the positive side of y-axis. Find the equation of the line.
P(5,3), Q(-4,7) and R(8,3) are he vertices of a traingles. Find the equation of the median of the traiangle from p.
Find the equation of a line that has Y-intercept 3 units and is perpendicular to the line joining (2, – 3) and (4, 2).
