Advertisements
Advertisements
Question
The fourth vertex D of a parallelogram ABCD, whose three vertices are A(–2, 3), B(6, 7) and C(8, 3), is ______.
Options
(0, 1)
(0, –1)
(–1, 0)
(1, 0)
Solution
The fourth vertex D of a parallelogram ABCD, whose three vertices are A(–2, 3), B(6, 7), and C(8, 3) is (0, –1).
Explanation:
Let the fourth vertex of the parallelogram, D ≡ (x4, y4) and L, M be the middle points of AC and BD, respectively,
Then, `L ≡ ((-2 + 8)/2, (3 + 3)/2) ≡ (3, 3)` and `M ≡ ((6 + x_4)/2, (7 + y_4)/2)` ...`["Since mid-point of a line segment having points" (x_1, y_1) "and" (x_2, y_2) = ((x_1 + x_2)/2, (y_1 + y_2)/2)]`

Since, ABCD is a parallelogram, therefore diagonals AC and BD will bisect each other.
Hence, L and M are the same points.
∴ 3 = `(6 + x_4)/2` and 3 = `(7 + y_4)/2`
⇒ 6 = 6 + x4 and 6 = 7 + y4
⇒ x4 = 0 and y4 = 6 – 7
∴ x4 = 0 and y4 = –1
Hence, the fourth vertex of the parallelogram is D = (x4, y4) = (0, –1).
RELATED QUESTIONS
Find the coordinates of the point which divides the line segment joining the points (6, 3) and (– 4, 5) in the ratio 3 : 2 internally.
In what ratio does the x-axis divide the line segment joining the points (2, –3) and (5, 6)? Also, find the coordinates of the point of intersection.
Prove that the points (–2, –1), (1, 0), (4, 3) and (1, 2) are the vertices of a parallelogram. Is it a rectangle ?
Prove that (4, – 1), (6, 0), (7, 2) and (5, 1) are the vertices of a rhombus. Is it a square?
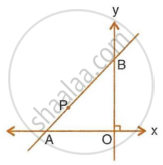
In the given figure, line APB meets the x-axis at point A and y-axis at point B. P is the point (−4, 2) and AP : PB = 1 : 2. Find the co-ordinates of A and B.
AB is a diameter of a circle with centre C = (–2, 5). If A = (3, –7), find
- the length of radius AC.
- the coordinates of B.
A (2, 5), B (-1, 2) and C (5, 8) are the vertices of triangle ABC. Point P and Q lie on AB and AC respectively, such that AP: PB = AQ: QC = 1: 2. Calculate the coordinates of P and Q. Also, show that 3PQ = BC.
If the points A(1, 2), O(0, 0), C(a, b) are collinear, then ______.
The points A(x1, y1), B(x2, y2) and C(x3, y3) are the vertices of ∆ABC. The median from A meets BC at D. Find the coordinates of the point D.
The points A(x1, y1), B(x2, y2) and C(x3, y3) are the vertices of ∆ABC. What are the coordinates of the centroid of the triangle ABC?
