Advertisements
Advertisements
Question
The height of a rectangular solid is 5 times its width and its length is 8 times its height. If the volume of the wall is 102.4 cm3, find its length.
Solution
Height of rectangular solid = 5 × width
and length = 8 × height = 8 × 5 × width = 40 × width
Volume = 102.4 cm3
Let width = w
Then height = 40w
and height = 5w
`therefore "w" xx 40"w" xx 5"w" = 102.4`
`"w"^3 = 102.4/(40 xx 5) = 0.512`
= `(0.8)^3`
∴ w = 0.8
∴ Length = 40w = `40 xx 0.8`
= 32 cm
APPEARS IN
RELATED QUESTIONS
The length, breadth and height of a room are 5 m, 4 m and 3 m respectively. Find the cost of white washing the walls of the room and the ceiling at the rate of Rs 7.50 per m2.
A small indoor greenhouse (herbarium) is made entirely of glass panes (including base) held together with tape. It is 30 cm long, 25 cm wide and 25 cm high. How much of tape is needed for all the 12 edges?
The length, breadth and height of a room are 5 m, 4 m and 3 m respectively. Find the cost
of white washing the walls of the room and the ceiling at the rate of Rs. 7.50 m2.
Three equal cubes are placed adjacently in a row. Find the ratio of total surface area of the new cuboid to that of the sum of the surface areas of the three cubes.
A 4 cm cube is cut into 1 cm cubes. Calculate the total surface area of all the small cubes.
The paint in a certain container is sufficient to paint on area equal to 9.375 m2. How manybricks of dimension 22.5 cm × 10 cm × 7.5 cm can be painted out of this container?
How many wooden cubical blocks of side 25 cm can be cut from a log of wood of size 3 m by 75 cm by 50 cm, assuming that there is no wastage?
How much clay is dug out in digging a well measuring 3 m by 2 m by 5 m?
What will be the height of a cuboid of volume 168 m3, if the area of its base is 28 m2?
A rectangular diesel tanker is 2 m long, 2 m wide and 40 cm deep. How many litres of diesel can it hold?
Find the surface area of a cuboid whose llength = 2 m, breadth = 4 m, height = 5 m .
Find the surface area of a cuboid whoselength = 3.2 m, breadth = 30 dm, height = 250 cm.
The dimensions of a cuboid are in the ratio 5 : 3 : 1 and its total surface area is 414 m2. Find the dimensions.
Find the area of the cardboard required to make a closed box of length 25 cm, 0.5 m and height 15 cm.
Find the length of the longest rod that can be placed in a room 12 m long, 9 m broad and 8 m high.
The external dimensions of a closed wooden box are 48 cm, 36 cm, 30 cm. The box is made of 1.5 cm thick wood. How many bricks of size 6 cm × 3 cm × 0.75 cm can be put in this box?
Find the edge of a cube whose surface area is 432 m2.
If the volumes of two cubes are in the ratio 8: 1, then the ratio of their edges is
A closed box is cuboid in shape with length = 40 cm, breadth = 30 cm and height = 50 cm. It is made of a thin metal sheet. Find the cost of metal sheet required to make 20 such boxes, if 1 m2 of metal sheet costs Rs. 45.
The length, breadth, and height of a room are 6 m, 5.4 m, and 4 m respectively. Find the area of :
(i) its four-walls
(ii) its roof.
A closed box measures 66 cm, 36 cm and 21 cm from outside. If its walls are made of metal-sheet, 0.5 cm thick; find :
(i) the capacity of the box ;
(ii) the volume of metal-sheet and
(iii) weight of the box, if 1 cm3 of metal weighs 3.6 gm.
The height of a circular cylinder is 20 cm and the diameter of its base is 14 cm. Find:
(i) the volume
(ii) the total surface area.
If the edge of a cube is 8 cm long, find its total surface area.
A closed box is made of wood 5 mm thick. The external length, breadth and height of the box are 21 cm, 13 cm and 11 cm respectively. Find the volume of the wood used in making the box.
The dimensions of a cuboidal box are 6 m × 400 cm × 1.5 m. Find the cost of painting its entire outer surface at the rate of ₹ 22 per m2.
Opposite faces of a cuboid are ______ in area.
The areas of any two faces of a cuboid are equal.
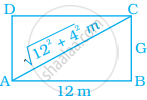
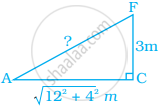
Find the length of the largest pole that can be placed in a room of dimensions 12 m × 4 m × 3 m.