Advertisements
Advertisements
Question
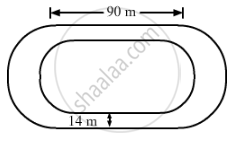
The inside perimeter of a running track shown in the figure is 400 m. The length of each of the straight portions is 90 m, and the ends are semicircles. If the track is 14 m wide everywhere, find the area of the track. Also, find the length of the outer boundary of the track.
Solution
Length of the inner curved portion (400 - 2 × 90) = 220 m
∴ Length of each inner curved path`=220/2=110 "m"`
Thus, we have:
⇒ πr = 110
`⇒ 22/7"r" = 110`
`=> "r" = (110xx7)/22`
⇒ r =35m
Inner radius = 35 m
Outer radius = (35 + 14) = 49 m
Area of track = {Area of the two rectangles [each(90 × 14)] + Area of the circular ring with R = 49 m and r = 35 m)}
`=(2xx90xx14)+22/7xx[(49)^2-(35)^2]`
`=2520 + 22/7xx(2401-1225)`
`= 2520 + 22/7xx1176`
= 2520 + 3696
= 6216 m2
Length of the outer boundary of the track
`=(2xx90+2xx22/7xx49)`
= 488 m
Therefore, the length of the outer boundary of the track is 488 m and the area of the track is 6216 sq. m.
APPEARS IN
RELATED QUESTIONS
In Fig. 7, are shown two arcs PAQ and PBQ. Arc PAQ is a part of circle with centre O and radius OP while arc PBQ is a semi-circle drawn on PQ ad diameter with centre M. If OP = PQ = 10 cm show that area of shaded region is `25(sqrt3-pi/6)cm^2`.

A square water tank has its side equal to 40m, there are 4 semicircular flower beds grassy plots all around it. Find the cost of turfing the plot at Rs 1.25/sq.m
The radii of two concentric circles are 19 cm and 16 cm respectively. The area of the ring enclosed by these circles is
Find the area and perimeter of the circle with the following :
Diameter = 77 cm
A wire when bent in the form of a square encloses an area of 484 cm2. If the same wire is bent into the form of a circle, find the area of the circle.
The circumference of a circle is equal to the perimeter of a square. The area of the square is 484 sq. m. Find the area of the circle.
A 7 m road surrounds a circular garden whose area is 5544 m2. Find the area of the road and the cost of tarring it at the rate of Rs.150 per sq m.
The diameter of a circular field is 56 m. Find its circumference and cost of fencing it at the rate of ₹80 per m. (Take π =`22/7`)
The cost of decorating the circumference of a circular lawn of a house at the rate of ₹ 55 per metre is ₹ 16940. What is the radius of the lawn?
The distance around a circle is its ______.
