Advertisements
Advertisements
Question
The circumference of a circle is equal to the perimeter of a square. The area of the square is 484 sq. m. Find the area of the circle.
Solution
Area of the square = 484 m2
Side of the square = `sqrt 484` = 22 m
Perimeter of the square = 4 x 22 = 88m
Perimeter of square = circumference of circle
2 π r = 88
r = `88 xx 7/22 xx 1/2`
r = 14 m
Radius of circle = 14 m
Area of the drde = πr2
`= 22/7 xx 14 xx 14`
= 616 m2
Hence, area of the circle = 616 m2
APPEARS IN
RELATED QUESTIONS
A race track is in the form of a ring whose inner circumference is 352 m, and the outer circumference is 396 m. Find the width of the track.
Saima wants to put a lace on the edge of a circular table cover of diameter 1.5 m. Find the length of the lace required and also find its cost if one meter of the lace costs ₹ 15. (Take π = 3.14)
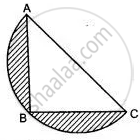
ABC is an isosceles right-angled triangle with ∠ABC = 90°. A semi-circle is drawn with AC as the diameter. If AB = BC = 7 cm, find the area of the shaded region. [Take π = 22/7]
Find the angle subtended at the centre of circle of radius ‘a’ cm by an arc of length
`(api)/4` 𝑐𝑚
A rectangular piece is 20m long and 15m wide from its four corners, quadrants of 3.5m radius have been cut. Find the area of remaining part.
Four equal circles each of radius a, touch each other. Show that area between them is `6/7a^2`
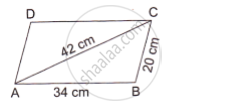
The adjacent sides of a parallelogram ABCD measure 34 cm and 20 cm, and the diagonal AC measures 42 cm. Find the area of the parallelogram.
The radius of a sector of a circle is 7 cm. If the measure of the arc of the sector is - three right angles; find the area of the sector in case.
Find the perimeter of a semicircular protractor whose diameter is 14 cm.
In the given figure, the shape of the top of a table is that of a sector of a circle with centre O and ∠AOB = 90°. If AO = OB = 42 cm,· then find the perimeter of the top of the table.
Four equal circles, each of radius a units, touch each other. Show that the area between them is `(6/7"a"^2)` sq units.
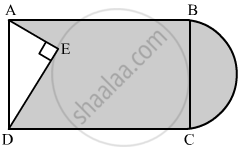
In the given figure, from a rectangular region ABCD with AB = 20 cm, a right triangle AED with AE = 9 cm and DE = 12 cm, is cut off. On the other end, taking BC as diameter, a semicircle is added on outside' the region. Find the area of the shaded region. [Use π = 3.14]
Find the diameter of the sphere for the following :
Volume = `72pi "cm"^3`
Using a ruler and compasses only:
(i) Construct a triangle ABC with the following data: AB = 3.5 cm, BC = 6 cm and ∠ ABC = 120°.
(ii) In the same diagram, draw a circle with BC as diameter. Find a point P on the circumference of the circle which is equidistant from AB and BC.
(iii) Measure ∠ BCP.
A ground is in the form of a circle whose diameter is 350 m. An athlete makes 4 revolutions. Find the distance covered by the athlete
Areas of two circles are equal. Is it necessary that their circumferences are equal? Why?
The area of a circular playground is 22176 m2. Find the cost of fencing this ground at the rate of Rs 50 per metre.
A circular pond is 17.5 m is of diameter. It is surrounded by a 2 m wide path. Find the cost of constructing the path at the rate of Rs 25 per m2.
The area of a square is 100 cm2. The circumference (in cm) of the largest circle cut of it is ______.
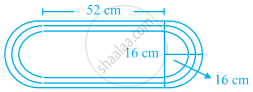
Ishika has designed a small oval race track for her remote control car. Her design is shown in the given figure. What is the total distance around the track? Round your answer to the nearest whole centimetre.